VECTORES en el Plano ↗️ Concepto, COORDENADAS y MÓDULO

Summary

In this video, Susi explains the fundamentals of vectors, focusing on how to calculate their coordinates and magnitude. Viewers learn how to represent vectors, how to determine the direction, and how to find the coordinates by subtracting the points' values. The concept of vector magnitude is also introduced using the Pythagorean theorem, showing how to calculate the length of a vector. The video emphasizes the importance of understanding both the vector’s direction and magnitude, and how reversing the vector doesn't affect its length. Susi uses clear examples to demonstrate these concepts, making them accessible for beginners.

Takeaways

• 😀 Vectors are segments that have both a direction and a magnitude.
• 😀 Vectors are typically represented with an arrow to indicate direction (e.g., A → B).
• 😀 Vectors can be defined using two points, and the direction can be reversed (e.g., from A to B or B to A).
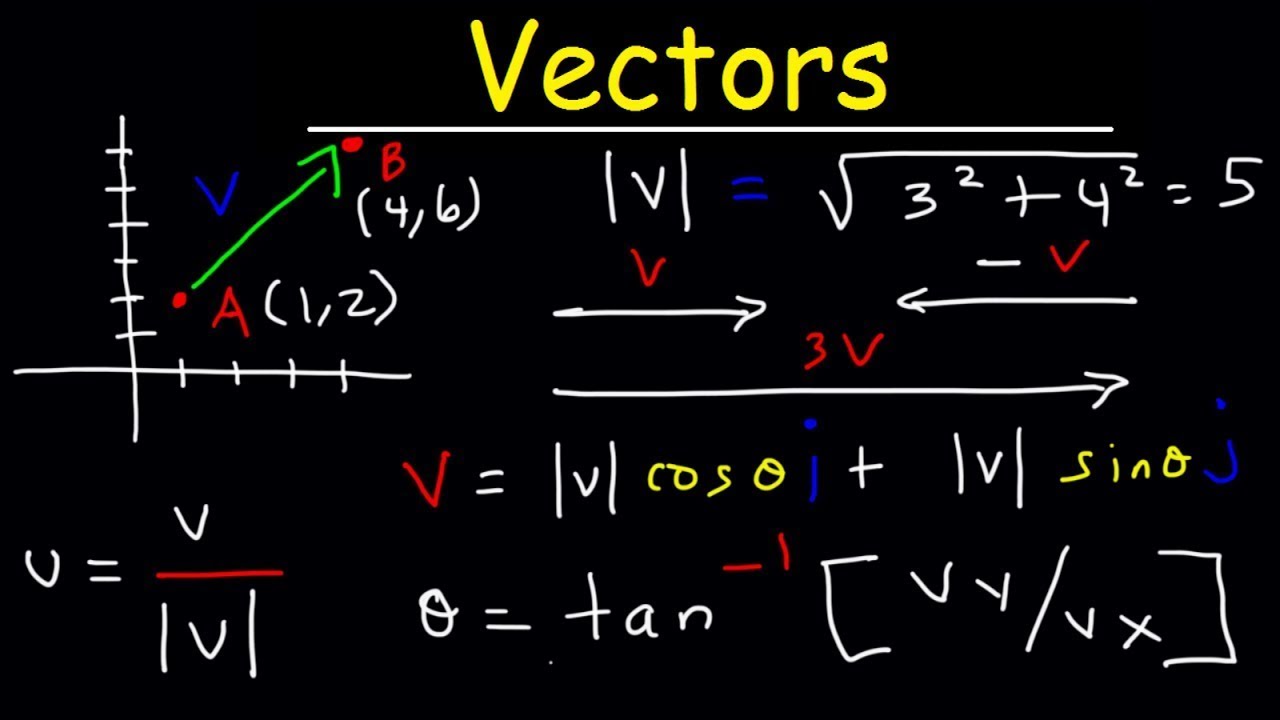
• 😀 To calculate the coordinates of a vector, subtract the coordinates of the origin point from the endpoint.
• 😀 When calculating the coordinates of a vector from A to B, subtract A's coordinates from B's (B - A).
• 😀 If a vector has negative coordinates, it means it moves in the opposite direction along the respective axis.
• 😀 The magnitude (module) of a vector is calculated using the Pythagorean theorem: √(x² + y²).
• 😀 The magnitude of a vector represents its length, regardless of direction (from A to B or B to A).
• 😀 The vector's direction and magnitude are independent of its starting and ending points, as long as the displacement remains the same.
• 😀 Understanding the concept of vectors visually (on a graph) makes calculations and comprehension easier, especially for beginners.
• 😀 The vector notation can be simplified by using variables like U, V, or W instead of writing A → B in long form.

Q & A

What is a vector?

A vector is a segment that has both direction and magnitude, representing movement or displacement between two points.

How do you represent a vector graphically?

A vector is typically represented by an arrow, where the starting point is the origin (0,0), and the arrow points to the destination or end point, such as point A.

What do the coordinates (3, 2) represent in a vector?

The coordinates (3, 2) represent the endpoint of the vector in a 2D coordinate system, starting from the origin (0,0). The vector moves 3 units along the x-axis and 2 units along the y-axis.

How do you calculate the coordinates of a vector from point A to point B?

To calculate the coordinates of a vector from point A to point B, subtract the coordinates of point A from point B. For example, if A is (1, 4) and B is (6, 6), the result is (6-1, 6-4) = (5, 2).

What is the difference between the vectors A to B and B to A?

The vector A to B indicates movement from point A to point B, while the vector B to A indicates the opposite direction, going from point B to point A. The coordinates will have opposite signs.

Why do vectors sometimes have negative coordinates?

Negative coordinates indicate movement in the opposite direction. For example, if a vector moves backward on the x-axis or downward on the y-axis, the coordinates will be negative.

How do you calculate the module (magnitude) of a vector?

The module of a vector is calculated using the Pythagorean theorem. For a vector with coordinates (x, y), the magnitude is √(x² + y²). For example, for the vector (5, 2), the module is √(5² + 2²) = √29.

What does the module of a vector represent?

The module of a vector represents its length or magnitude, showing how much the vector 'measures' in terms of distance between the origin and the end point.

Does the direction of a vector affect its magnitude?

No, the magnitude (module) of a vector does not change with its direction. A vector from A to B will have the same magnitude as a vector from B to A, as long as the distance remains the same.

What is the significance of using arrows over vector labels like A to B or B to A?

The arrow over the vector label indicates that it is a vector, which is different from a simple point. The direction of the arrow shows the vector's direction, while the label helps identify the vector's starting and ending points.