Fungsi Rasional kelas XI Matematika Tingkat Lanjut (Kurikulum Merdeka)

Summary

This video tutorial explores rational and irrational functions, focusing on rational functions in the form of fractions where the numerator and denominator are both mathematical functions. It explains the key requirement that the denominator cannot equal zero to avoid undefined values. Examples of rational functions and their respective conditions are provided. The tutorial also covers the concepts of vertical and horizontal asymptotes, showing how to identify them through graphical analysis. Viewers learn how to determine the locations of both vertical and horizontal asymptotes from function graphs, using clear examples to guide understanding.

Takeaways

• 😀 Rational functions are fractions of polynomials, where the numerator and denominator are polynomials, and the denominator must not equal zero.
• 😀 An example of a rational function is f(x) = (2x + 3) / (4x + 5), where the denominator must not be zero.
• 😀 Rational functions can have vertical asymptotes, which occur when the function approaches infinity as x approaches certain values.
• 😀 Vertical asymptotes are determined by finding when the denominator of the rational function equals zero and solving for x.
• 😀 Horizontal asymptotes represent the behavior of the function as x approaches infinity, where the function approaches a constant value.
• 😀 For a function like f(x) = (3x^2 + 4) / (3x - 3), the condition is that 3x - 3 should not be zero to avoid undefined behavior.
• 😀 The general condition for rational functions is that the denominator must not equal zero to prevent undefined values.
• 😀 Rational functions can be in different forms, such as linear over linear or polynomial over polynomial.
• 😀 Asymptotes help describe the behavior of the graph of a rational function, indicating where the graph approaches but never reaches certain values.
• 😀 In practice, to find vertical asymptotes, set the denominator equal to zero, and to find horizontal asymptotes, look at the behavior as x approaches infinity.

Q & A

What is a rational function?

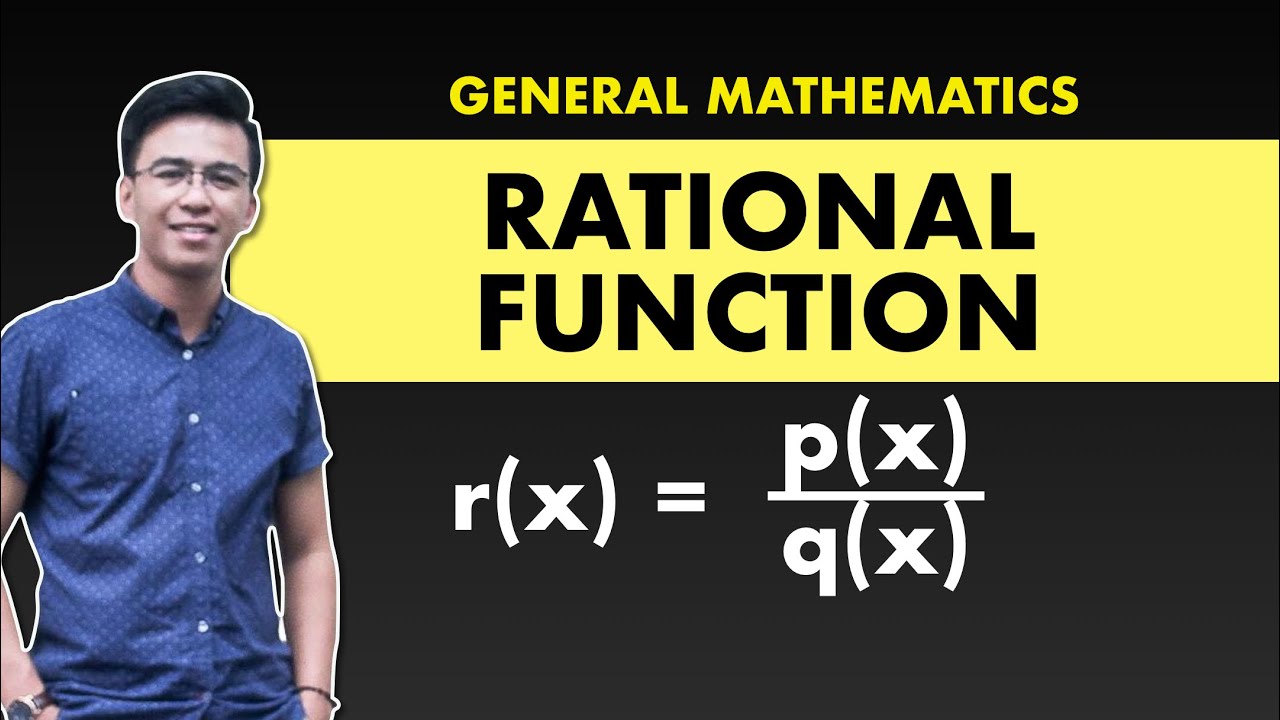
A rational function is a function that can be expressed as the ratio of two polynomials. It takes the form f(x) = P(x) / Q(x), where both P(x) and Q(x) are polynomials and Q(x) is not equal to zero.

What is an example of a rational function?

An example of a rational function is f(x) = (3x^2 + 4) / (x - 3). This function consists of a polynomial in the numerator and denominator.

What is the significance of the denominator in a rational function?

The denominator in a rational function is crucial because it determines where the function may be undefined. The function is undefined where the denominator equals zero.

What happens if the denominator of a rational function equals zero?

If the denominator equals zero, the function is undefined at that point. This typically results in a vertical asymptote in the graph of the function.

What is a vertical asymptote?

A vertical asymptote is a vertical line x = a, where the function’s value approaches infinity (or negative infinity) as x approaches the value 'a' from either side.

How do you find the vertical asymptote of a rational function?

To find the vertical asymptote of a rational function, set the denominator equal to zero and solve for x. The solution gives the location of the vertical asymptote.

What is a horizontal asymptote?

A horizontal asymptote is a horizontal line y = b, where the function’s value approaches the constant 'b' as x approaches positive or negative infinity.

How is a horizontal asymptote determined for a rational function?

The horizontal asymptote is determined by comparing the degrees of the numerator and denominator polynomials. If the degrees are the same, the horizontal asymptote is the ratio of the leading coefficients. If the degree of the numerator is greater, there is no horizontal asymptote.

What happens to a rational function's graph when it approaches a horizontal asymptote?

When a rational function’s graph approaches a horizontal asymptote, the function's values approach a constant value as x moves towards positive or negative infinity, causing the graph to level off.

How can we identify the vertical and horizontal asymptotes from a graph of a rational function?

From the graph of a rational function, vertical asymptotes can be identified where the graph tends toward infinity (upward or downward), and horizontal asymptotes can be identified by observing where the graph levels off as x approaches large positive or negative values.