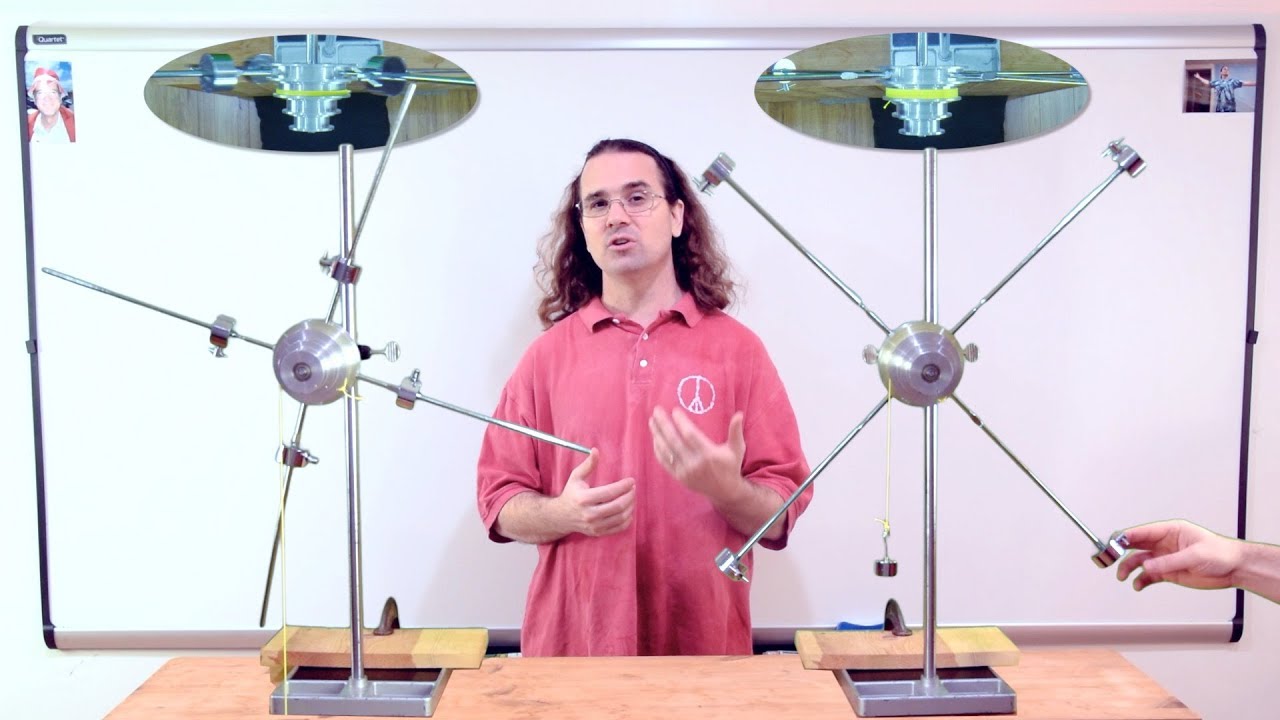
Introductory Moment of Inertia and Rotational Kinetic Energy Problem

Summary

In this lesson, students explore the concepts of moment of inertia and rotational kinetic energy through a system of three rotating masses. The class calculates the moment of inertia using mass and distance from the axis of rotation, then determines the rotational kinetic energy using the appropriate formulas. Along the way, they address key questions about units and the relationship between angular velocity and moment of inertia, learning the importance of SI units for accurate results. The session concludes with a comparison of the system's rotational energy to its gravitational potential energy, helping contextualize the calculations.

Takeaways

• 😀 The moment of inertia of a system of particles is calculated by summing the product of each mass and the square of its distance from the axis of rotation.
• 😀 The radius and the distance from the axis of rotation are the same in this example, although they are not the same as the length of the string.
• 😀 The moment of inertia does not depend on the angular velocity of the objects in the system.
• 😀 The reason angular velocity was included in the problem was to calculate the rotational kinetic energy of the system.
• 😀 Rotational kinetic energy is calculated using the formula (1/2) * moment of inertia * (angular velocity)^2.
• 😀 To work in SI units, mass is converted from grams to kilograms, distance is converted from centimeters to meters, and angular velocity is converted from revolutions per minute to radians per second.
• 😀 In SI units, the correct unit for rotational kinetic energy is joules, which requires the moment of inertia in kilogram meters squared and angular velocity in radians per second.
• 😀 The final rotational kinetic energy was found to be 6.7 x 10^-2 joules for the system of three rotating masses.
• 😀 The units for rotational kinetic energy were verified and shown to be correct by simplifying the units through the process.
• 😀 For comparison, the potential energy of the system was considered by assuming the masses are 1 meter above the ground, with a gravitational potential energy of 200 millijoules each.

Q & A

What is the main objective of the lesson in this transcript?

The main objective of the lesson is to determine the moment of inertia of a system of three masses rotating in a circle.

Why was angular velocity not used to calculate the moment of inertia?

The moment of inertia depends only on the mass and the distance from the axis of rotation, not on the angular velocity. Angular velocity is not required for calculating the moment of inertia.

How did Billy simplify the expression for the moment of inertia?

Billy simplified the expression by recognizing that all the masses are the same and the distances from the axis of rotation are the same, allowing him to use a common variable for mass and distance. This led to the formula: Moment of inertia = 3 * m * r^2.

What was the key concept that Mr.p emphasized about the radius and distance from the axis of rotation?

Mr.p emphasized that, in this example, the radius and the distance from the axis of rotation are the same thing. However, the length of the string is not the same as the radius.

What did Bobby initially question about the inclusion of angular velocity in the problem?

Bobby questioned why angular velocity was included in the problem when it was not needed for calculating the moment of inertia.

What is the formula for rotational kinetic energy?

The formula for rotational kinetic energy is: Rotational kinetic energy = 1/2 * Moment of inertia * (angular velocity)^2.

What did Bo calculate for the rotational kinetic energy of the system?

Bo calculated the rotational kinetic energy to be 6.1 × 10^7, or approximately 6.1 × 10^7, in the incorrect units, before they corrected them to SI units.

What was the issue with the units of the rotational kinetic energy initially?

The initial units were in grams, centimeters squared, and revolutions squared per minute squared, which are not appropriate for energy calculations. The units needed to be converted to SI base units.

How did Bobby correct the units for the moment of inertia and rotational kinetic energy?

Bobby converted the mass to kilograms, the radius to meters, and the angular velocity to radians per second, which led to the correct SI units for the moment of inertia and rotational kinetic energy.

What was the final rotational kinetic energy of the system in joules?

The final rotational kinetic energy of the system was 6.7 × 10^-2 joules, or 0.067 joules, after converting the units to SI base units.