Double Angle Identities & Formulas of Sin, Cos & Tan - Trigonometry
Summary
TLDRThis video explains the key double angle formulas in trigonometry, providing clear step-by-step derivations and applications for sine, cosine, and tangent. It covers the formulas for sine (2θ = 2sin(θ)cos(θ)), cosine (2θ = cos²(θ) - sin²(θ)), and tangent (2θ = 2tan(θ) / (1 - tan²(θ))). Through detailed examples using right triangles, the video demonstrates how to calculate these double angle values for different angles. Viewers will gain a deeper understanding of how to apply these formulas in solving problems involving trigonometric functions.
Takeaways
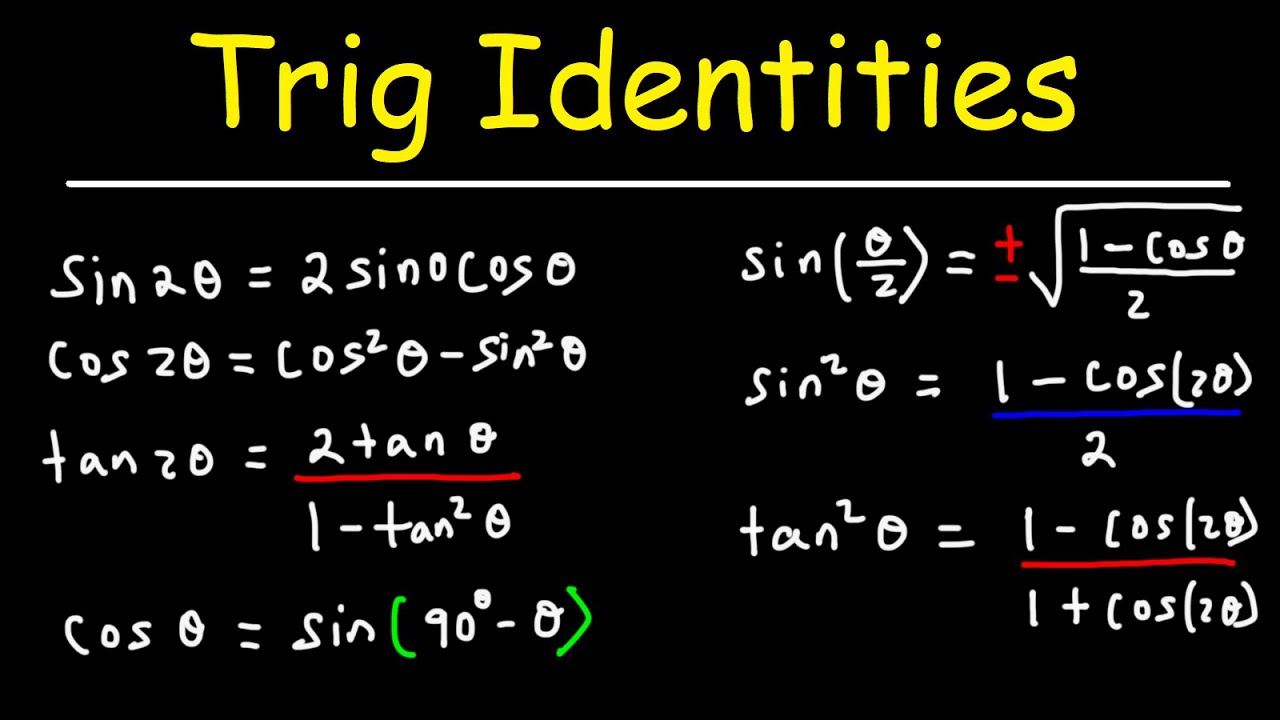
- 😀 The sine double angle formula is: sin(2θ) = 2 sin(θ) cos(θ).
- 😀 The cosine double angle formula is: cos(2θ) = cos²(θ) - sin²(θ). It can also be written as cos(2θ) = 2 cos²(θ) - 1 or cos(2θ) = 1 - 2 sin²(θ).
- 😀 The tangent double angle formula is: tan(2θ) = (2 tan(θ)) / (1 - tan²(θ)).
- 😀 To apply the sine double angle formula, simply multiply the sine and cosine of the angle and double the result.
- 😀 The cosine double angle formula can be derived using the sum of angles identity for cosine: cos(α + β) = cos(α) cos(β) - sin(α) sin(β).
- 😀 Tangent of a double angle can be calculated using the quotient identity for tangent: tan(2θ) = sin(2θ) / cos(2θ).
- 😀 Trigonometric identities simplify the process of finding values for double angles in trigonometric functions.
- 😀 When given values for sine, cosine, and tangent of an angle, you can easily find the values for sine, cosine, and tangent of double angles.
- 😀 For example, with sin(θ) = 3/5, cos(θ) = 4/5, the sine of the double angle sin(2θ) = 24/25, cosine of the double angle cos(2θ) = 7/25, and tangent of the double angle tan(2θ) = 24/7.
- 😀 The formulas help simplify complex expressions, such as 2 sin(75°) cos(75°) becoming sin(150°) = 1/2, and cos²(15°) - sin²(15°) simplifying to cos(30°) = √3/2.
Q & A
What is the double angle formula for sine?
-The double angle formula for sine is given by: sine(2θ) = 2 sine(θ) cosine(θ).
How is the double angle formula for cosine derived?
-The double angle formula for cosine is derived using the sum of angles formula for cosine: cosine(α + β) = cosine(α) cosine(β) - sine(α) sine(β). When α and β are both equal to θ, this becomes cosine(2θ) = cosine²(θ) - sine²(θ).
What is the double angle formula for tangent?
-The double angle formula for tangent is: tangent(2θ) = 2 tangent(θ) / (1 - tangent²(θ)).
How do you find sine(2θ) when sine(θ) = 3/5?
-To find sine(2θ), use the formula sine(2θ) = 2 sine(θ) cosine(θ). Given sine(θ) = 3/5 and cosine(θ) = 4/5, you substitute these values into the formula: sine(2θ) = 2 * (3/5) * (4/5) = 24/25.
How do you calculate cosine(2θ) when sine(θ) = 3/5 and cosine(θ) = 4/5?
-To calculate cosine(2θ), use the formula cosine(2θ) = cosine²(θ) - sine²(θ). With cosine(θ) = 4/5 and sine(θ) = 3/5, substitute into the formula: cosine(2θ) = (4/5)² - (3/5)² = 16/25 - 9/25 = 7/25.
What is the value of tangent(2θ) when sine(2θ) = 24/25 and cosine(2θ) = 7/25?
-To find tangent(2θ), use the quotient identity: tangent(2θ) = sine(2θ) / cosine(2θ). With sine(2θ) = 24/25 and cosine(2θ) = 7/25, tangent(2θ) = (24/25) / (7/25) = 24/7.
Why is the first method of finding tangent(2θ) easier than the second method?
-The first method, using sine(2θ) and cosine(2θ) directly, is simpler because it involves straightforward division of the two values. The second method involves a more complex formula and additional steps, including squaring and multiplying fractions.
How do you solve for sine(2θ) when cosine(θ) = 5/13 in quadrant IV?
-First, draw a right triangle in quadrant IV where cosine(θ) = adjacent/hypotenuse = 5/13. Using the Pythagorean theorem, find that sine(θ) = -12/13. Then, use the formula sine(2θ) = 2 sine(θ) cosine(θ) to calculate sine(2θ) = 2 * (-12/13) * (5/13) = -120/169.
How is cosine(2θ) calculated when cosine(θ) = 5/13 and sine(θ) = -12/13 in quadrant IV?
-To find cosine(2θ), use the formula cosine(2θ) = cosine²(θ) - sine²(θ). With cosine(θ) = 5/13 and sine(θ) = -12/13, substitute these values: cosine(2θ) = (5/13)² - (-12/13)² = 25/169 - 144/169 = -119/169.
What is the exact value of the expression 2 sine(75°) cosine(75°)?
-Using the double angle formula for sine, 2 sine(75°) cosine(75°) = sine(2 * 75°) = sine(150°). Since sine(150°) = 1/2, the exact value of the expression is 1/2.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тариф5.0 / 5 (0 votes)