Calculus AB/BC – 1.4 Estimating Limit Values from Tables
Summary
TLDRIn this calculus lesson, Mr. Bean introduces the concept of finding limits using a table of values and a graphing calculator, specifically the TI-84 Plus CE. He demonstrates how to approximate limits by inputting values close to a given point and discusses the importance of using a calculator for accuracy. The lesson covers the use of table setup for precise values, function notation for more accurate calculations, and emphasizes the need for rounding or truncating to three decimal places for AP exam standards. Mr. Bean also touches on the continuity and increasing nature of functions, concluding with a practical example of finding the cosine of a limit.
Takeaways
- 📚 The lesson is about finding limits in calculus, emphasizing the importance of using a calculator, especially a graphing calculator, for accuracy and efficiency.
- ⚠️ A warning is given that a calculator will be necessary for the lesson, with a suggestion to use a graphing calculator for the best results.
- 📈 The concept of limits is illustrated through a table of values, showing how Y-values approach a certain number as X gets closer to a specific value.
- 📉 The graphical representation of limits is discussed, with an example of how the limit is determined when X approaches -4, resulting in a Y-value approaching 2.5.
- 🔢 The use of a TI-84 Plus CE calculator is demonstrated to fill in a table of values for evaluating limits, with the function plugged into the calculator for quick computation.
- 🛠 Two methods are shown for using the calculator: the table of values and function notation, with the latter providing more accurate results due to less rounding error.
- 📉 The function notation method is highlighted as particularly useful for future problems, allowing for quick and accurate input of values into the function.
- 📌 The importance of setting the calculator to radian mode for trigonometric functions in calculus is emphasized, as opposed to degree mode.
- 📝 The lesson concludes with an example of how to calculate the limit of a function as X approaches a certain value, using the cosine function and the calculator for precise results.
- ✂️ The importance of truncating or rounding to three decimal places for exam answers is stressed, as this is a requirement for the AP exam.
- 🎓 The lesson ends with a reminder to practice the skills learned for the mastery check, highlighting the significance of precision and accuracy in calculus.
Q & A
What is the main topic of the calculus lesson presented by Mr. Bean?
-The main topic of the lesson is finding limits in calculus using a table of values and a graphing calculator.
Why is a calculator recommended for this lesson?
-A calculator is recommended because it helps in evaluating limits by plugging in values close to the point of interest, and a graphing calculator is particularly useful for its table of values feature.
What does the limit represent in the context of the lesson?
-The limit represents the value that the function's output (Y-value) approaches as the input (X) gets arbitrarily close to a certain point.
How does the table of values help in understanding limits?
-The table of values helps by showing the Y-values for X-values that are very close to the point of interest, illustrating what the Y-value is approaching as X approaches that point.
What is the significance of the graphical representation in the lesson?
-The graphical representation visually shows the behavior of the function as X approaches a certain value, helping to understand the concept of limits and how they are approached.
Why is it necessary to use function notation on a calculator for more accuracy?
-Function notation on a calculator allows for more precise input of values into the function, providing more accurate results with additional decimal places compared to the table of values.
What is the purpose of setting the calculator to radian mode when dealing with trigonometric functions in calculus?
-Radian mode is necessary because calculus and physics typically use radians for trigonometric functions, as opposed to degrees.
How does the statement about the function being continuous and increasing affect the interpretation of the limit?
-The statement ensures that there are no breaks or decreases in the graph, which confirms that the Y-values are consistently approaching a certain number from both sides of X.
What is the importance of using three decimal places when solving problems for the 8P exam?
-Using three decimal places is a requirement for the 8P exam to ensure precision in the answers, and it helps the graders to quickly assess the accuracy of the solutions.
What are the two methods mentioned in the script for dealing with decimal places in answers?
-The two methods are rounding the answer to three decimal places or truncating the answer, which involves writing out three decimal places and stopping.
How does the script emphasize the importance of understanding the concept of limits in calculus?
-The script emphasizes the importance by providing a step-by-step approach to finding limits using tables and calculators, and by explaining the significance of each step in the process.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示
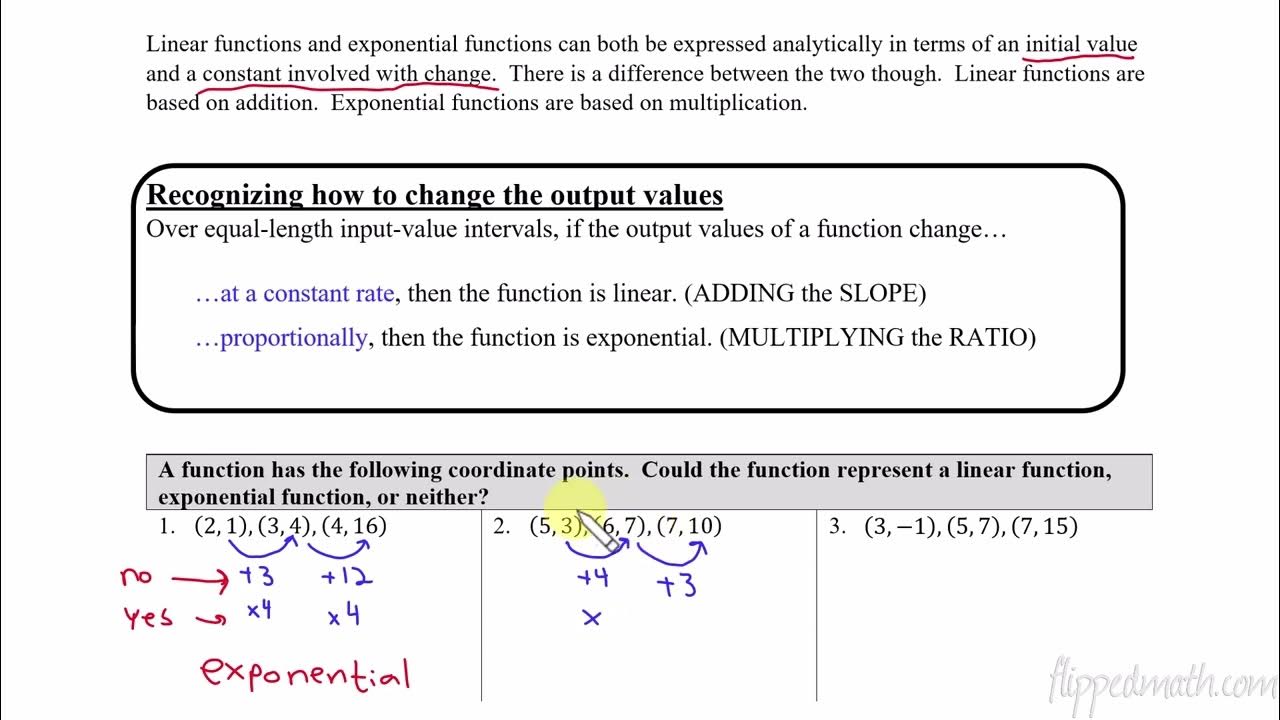
AP Precalculus – 2.2 Change in Linear and Exponential Functions
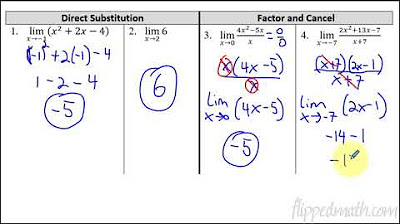
Calculus AB/BC – 1.6 Determining Limits Using Algebraic Manipulation

Calculus AB/BC – 1.10 Exploring Types of Discontinuities

Calculus AB/BC – 2.1 Defining Average and Instantaneous Rate of Change at a Point

Calculus BC – 10.12 Lagrange Error Bound

Prereq 13: Complex Numbers
5.0 / 5 (0 votes)
