Matematika SMP - Pemfaktoran Aljabar (1) - Rumus Dasar, Rumus Jumlah dan Selisih
Summary
TLDRThis video tutorial provides a comprehensive guide to multiplication and factoring in algebra, focusing on special multiplication formulas like the distributive property and the process of factoring expressions. It covers step-by-step examples of how to handle polynomials with various terms, including identifying common factors, applying the difference of squares, and factoring out common terms. The tutorial includes clear explanations of both the forward and reverse processes, with practical examples for students to follow along and master algebraic concepts.
Takeaways
- 😀 The first formula discussed is the distributive property, which involves multiplying a factor with the terms inside parentheses (e.g., a(b + c - d) = ab + ac - ad).
- 😀 The process of factoring is introduced as the reverse of multiplication. For example, from terms like ab + ac, you can factor out the common 'a' to get a(b + c).
- 😀 When performing multiplication of polynomials, it’s important to multiply each term in one binomial with every term in the other binomial.
- 😀 The transcript includes examples where students learn how to calculate powers of variables, like x² × x² = x⁴ or x² × x = x³, emphasizing proper exponentiation.
- 😀 The importance of recognizing common factors in expressions is highlighted, such as extracting the greatest common factor (GCF) before simplifying a polynomial.
- 😀 A key example shows how to factor expressions with multiple variables by looking for common factors, like in '3ab + 6ab² - 9ab' where you factor out 3ab.
- 😀 When dealing with complex terms, sometimes factoring involves grouping terms in pairs or triplets to identify common factors, as demonstrated in some of the provided examples.
- 😀 Special formulas are introduced for expressions like (a + b)(a - b), which simplifies to a² - b², illustrating the difference of squares formula.
- 😀 Students are encouraged to practice simplifying and factoring polynomials by breaking down each term systematically, as shown in the examples involving multiple variables and constants.
- 😀 The lesson ends with the application of the difference of squares formula in more complex scenarios, reinforcing how to factor and expand terms using the a² - b² rule.
Q & A
What is the distributive property in multiplication and how is it used in factoring?
-The distributive property is a fundamental rule in multiplication where you multiply a number outside the parentheses with each term inside the parentheses. For example, in the expression a(b + c - d), you distribute a to each term inside the parentheses, resulting in ab + ac - ad.
How does factoring reverse the process of multiplication?
-Factoring is essentially the reverse of multiplication. While multiplication expands an expression, factoring involves looking for common terms and rewriting the expression in a simpler form. For instance, in the expression ab + ac + ad, you can factor out the common 'a' to get a(b + c + d).
What is the result when multiplying terms with exponents, like x² * x²?
-When multiplying terms with exponents, you add the exponents. For example, x² * x² equals x^(2+2) = x⁴.
How do you handle a multiplication problem with both constants and variables, like 2x²y * (x² - 3xy - 4y³)?
-In such problems, you distribute 2x²y to each term inside the parentheses. This means multiplying 2x²y by each term separately: 2x²y * x², 2x²y * -3xy, and 2x²y * -4y³. You then simplify each product accordingly.
What is the process for factoring an expression like 3a²b + 6ab² - 9ab?
-To factor this expression, you first identify the greatest common factor (GCF). The GCF here is 3ab. Once factored out, the expression becomes 3ab(a + 2b - 3).
How do you factor a quadratic expression like 2ax - 10y - 3bx + 15y?
-You first factor out the common terms from the pairs of terms. For example, from 2ax - 10y, you can factor out 2a, leaving x - 5y. From -3bx + 15y, you can factor out -3b, leaving x - 5y. Finally, you factor out the common binomial (x - 5y) and the result is (x - 5y)(2a - 3b).
What is the special formula for the difference of squares, and how is it applied?
-The difference of squares formula is a² - b² = (a + b)(a - b). This formula is used when you have an expression where two squared terms are subtracted, such as x² - 9. This can be factored as (x + 3)(x - 3).
Can the difference of squares formula be applied to more complex terms like 3a³ - 15a?
-Yes, the difference of squares formula can be applied when the terms can be factored first. In this case, 3a³ - 15a can be factored as 3a(a² - 5), and the formula can then be used if you have a difference of squares, such as a² - b².
How do you handle expressions with multiple variables and exponents, such as 6a² + 8ac + 9ab + 12bc?
-In expressions with multiple variables and exponents, you first identify common factors in the terms. For example, in 6a² + 8ac, you can factor out 2a, and in 9ab + 12bc, you can factor out 3b. The factored expression will be (2a + 3b)(3a + 4c).
What steps should you take when factoring an expression like x² - 81?
-The expression x² - 81 is a difference of squares. You apply the difference of squares formula: x² - 81 = (x + 9)(x - 9). This works because 81 is a perfect square, and the formula can be applied directly.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

Adding and Subtracting Rational Algebraic Expressions Part 2 - Grade 8 Mathematics

LE COURS : Factorisations - Troisième

FACTORIZACIÓN POR FACTOR COMÚN Super facil - Para principiantes
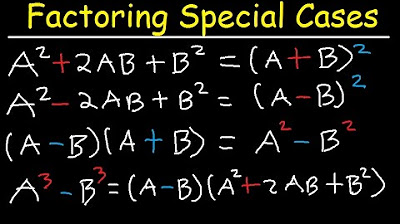
Factoring Binomials & Trinomials - Special Cases

MULTIPLICATION AND DIVISION OF RATIONAL ALGEBRAIC EXPRESSIONS || GRADE 8 MATHEMATICS Q1

Common Monomial Factoring - Polynomial Factoring - Grade 8 Math
5.0 / 5 (0 votes)
