HUBUNGAN ANTARA SUDUT PUSAT DAN SUDUT KELILING LINGKARAN ~ Materi Matematika Kelas 8 Semester 2
Summary
TLDRIn this educational video, the presenter explains the relationship between central and inscribed angles in a circle, specifically for 8th grade mathematics. The video covers the concepts of central angles, where the vertex is at the circle's center, and inscribed angles, where the vertex is on the circumference. Through examples and detailed step-by-step solutions, the presenter highlights how the central angle is always twice the size of the inscribed angle that subtends the same arc. The video also includes problem-solving techniques and encourages viewers to engage by liking, commenting, and subscribing.
Takeaways
- 😀 Central angle: An angle whose vertex is at the center of the circle, and whose arms are formed by two radii.
- 😀 Inscribed angle: An angle whose vertex lies on the circle, and whose arms are formed by two chords of the circle.
- 😀 The central angle and the inscribed angle subtending the same arc are related by the formula: Central angle = 2 × Inscribed angle.
- 😀 Conversely, the inscribed angle is half the central angle: Inscribed angle = ½ × Central angle.
- 😀 A central angle has its vertex at the center of the circle, while an inscribed angle has its vertex on the circumference.
- 😀 When two angles (one central and one inscribed) subtend the same arc, their values are related by the mentioned formulas.
- 😀 If multiple inscribed angles subtend the same arc, they all have the same measure.
- 😀 In a problem, first analyze the given angle (whether central or inscribed) and its relation to the subtended arc.
- 😀 Example: If an inscribed angle is given as 38°, and it subtends an arc, the central angle that subtends the same arc will be 76°.
- 😀 The formulas for central and inscribed angles only apply when both angles subtend the same arc.
- 😀 Always ensure that the central and inscribed angles are compared with the correct corresponding arcs to apply the formulas correctly.
Q & A
What is the topic of this video?
-The video discusses mathematics material for Grade 8 students, focusing on circles, specifically the relationship between central angles and inscribed angles.
What is a central angle in a circle?
-A central angle is an angle whose vertex is at the center of the circle, and whose arms (or sides) are the radii of the circle.
What is an inscribed angle in a circle?
-An inscribed angle is an angle whose vertex is on the circumference of the circle, and whose arms are chords (or segments) of the circle.
How is a central angle different from an inscribed angle?
-A central angle has its vertex at the center of the circle, whereas an inscribed angle has its vertex on the circumference of the circle.
What is the relationship between a central angle and an inscribed angle?
-The relationship is that the measure of a central angle is always twice the measure of an inscribed angle that subtends the same arc. The formula is: central angle = 2 × inscribed angle.
Can the formula for the central angle and inscribed angle relationship be applied to any angles?
-Yes, the formula applies when both the central and inscribed angles subtend the same arc of the circle.
What is the rule for angles subtended by the same arc?
-When two or more angles subtend the same arc, the central angle will be twice the size of the inscribed angle.
What is the central angle formed by the radii OA and OB in the example?
-In the example, the angle formed by the radii OA and OB is a central angle, which is the angle at the center of the circle.
How can you calculate the central angle if the inscribed angle is given?
-To calculate the central angle, simply multiply the inscribed angle by 2. For example, if the inscribed angle is 38°, the central angle would be 76°.
What is the correct approach for solving a problem involving central and inscribed angles?
-First, identify the type of angles (central or inscribed), and if they subtend the same arc, use the formula: central angle = 2 × inscribed angle. Then, apply this relationship to find the unknown angles.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

Materi Lingkaran dan Busur Lingkaran Bab 2 Matematika Umum Kelas 11 SMA Kurikulum Merdeka

Materi Lingkaran, Unsur Lingkaran dan Hubungan Sudut Pusat Sudut Keliling Kelas XI Kur Merdeka

Lingkaran [Part 2] - Sudut Pusat dan Sudut Keliling

Lingkaran kelas 11 / Video Teorema Lingkaran
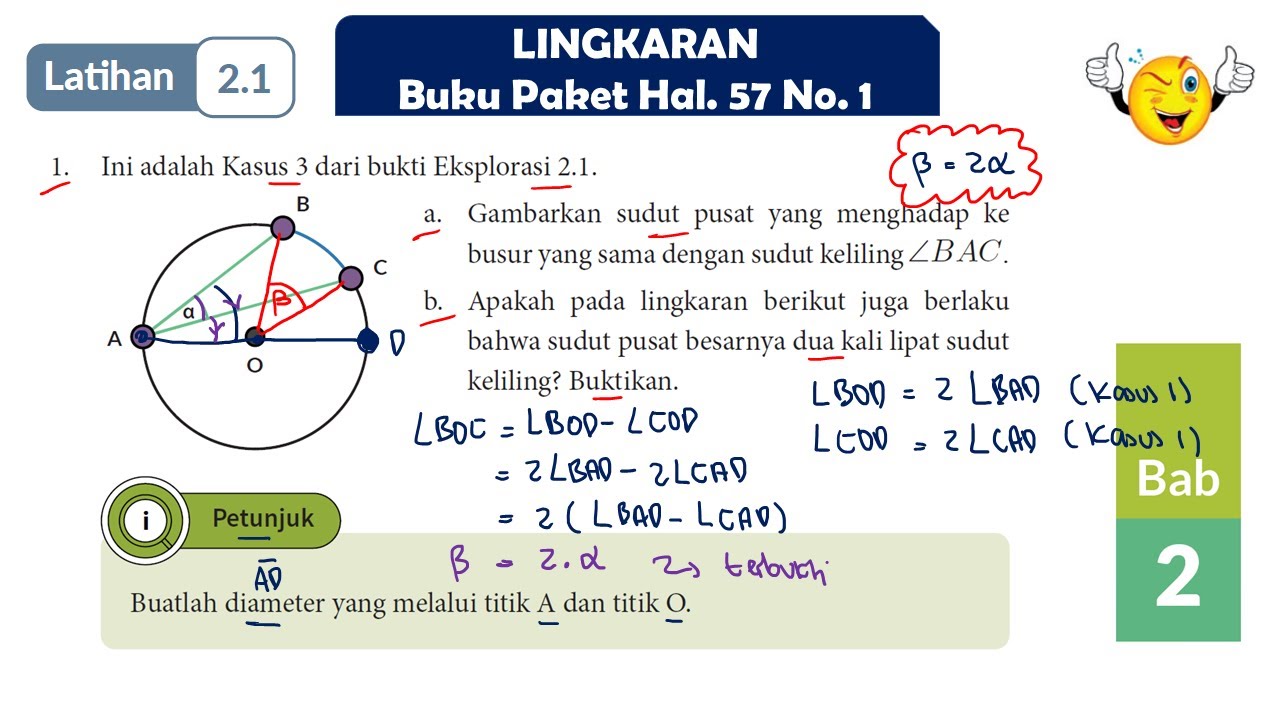
LATIHAN 2.1 NO 1 SUDUT PUSAT SUDUT KELILING MATEMATIKA SMA KELAS 11 #kurikulummerdeka #matematikasma

Perhatikan gambar lingkaran dengan pusat O! Jika besar sudut AOD=80, besar sudut ACB=.... C D O A...
5.0 / 5 (0 votes)
