Relasi & Fungsi (1) - Relasi, Pengertian Relasi, Contoh Relasi - Matematika SMP
Summary
TLDRIn this video, the host explains the concept of functions in mathematics, particularly focusing on the relationships between sets. The video covers different types of functions, including relations, mappings (functions), and one-to-one correspondence. Viewers learn about arrow diagrams, Cartesian diagrams, and ordered pair sets as ways to represent functions. Key concepts like domain, codomain, and range are discussed in detail. Through examples, the host demonstrates how to determine these components and how to depict them graphically. The video provides a clear and engaging explanation of basic function theory for beginners.
Takeaways
- 📚 Functions in math represent relationships between two sets.
- 📊 Three types of relationships: relations, functions (mappings), and one-to-one correspondences.
- 🔄 Functions can be represented in different forms: arrow diagrams, Cartesian diagrams, and ordered pairs.
- 📈 Domains represent the set of all possible inputs, while codomains represent all possible outputs.
- 🏹 Arrow diagrams must show direction, indicating the mapping of elements from one set to another.
- 🔢 In ordered pairs, elements from the domain are mapped to elements in the codomain.
- 📏 Be mindful of the details like arrows and brackets in diagrams to avoid mistakes.
- 📝 In relations, multiple outputs for the same input are allowed, unlike in functions.
- 📐 Cartesian diagrams use the X-axis for the domain and the Y-axis for the codomain to plot relations.
- 🔍 Understanding the relation is key to determining if it’s a function, mapping, or one-to-one correspondence.
Q & A
What is the concept of a function in mathematics?
-A function in mathematics is a way to describe a relationship between two sets, where each element in one set is related to one or more elements in another set.
What are the three types of functions introduced in this video?
-The three types of functions introduced are relations, mappings (also called functions), and one-to-one correspondences.
How is a function represented using an arrow diagram?
-In an arrow diagram, two sets (for example, A and B) are represented with arrows showing which elements in set A are related to elements in set B.
What is a Cartesian diagram, and how is it used to show functions?
-A Cartesian diagram, or Cartesian coordinate system, displays two sets on perpendicular axes (typically x for set A and y for set B), allowing the function's pairs to be represented as points on a grid.
What are ordered pairs, and how are they used in functions?
-Ordered pairs are sets of two numbers written in the form (x, y) that represent the relationship between elements in two sets; in functions, they indicate how an element from set A is paired with an element in set B.
What are domain, codomain, and range in a function?
-The domain is the set of all starting values in a function, the codomain is the set of all potential outputs, and the range is the set of actual outputs that are the result of the function’s mapping.
How do we determine the domain, codomain, and range in an arrow diagram?
-In an arrow diagram, the domain includes all elements in the starting set (left side), the codomain includes all elements in the ending set (right side), and the range consists of only those codomain elements with arrows pointing to them.
What differentiates a relation from a function?
-A relation has no strict rules for pairing elements; any element in the first set can relate to any element in the second set. A function, however, has specific rules, such as each element in the domain only pairing with one element in the codomain.
How can we identify if a relation is one-to-one correspondence?
-A relation is a one-to-one correspondence if each element in the domain is paired with exactly one unique element in the codomain, and each element in the codomain is paired with only one unique element in the domain.
What is the importance of arrows in an arrow diagram, and what should be considered?
-Arrows in an arrow diagram show the direction of the relationship between sets, and it's important to ensure that the arrows are placed correctly to avoid misinterpretation, especially in terms of the domain and codomain.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

APRENDA FUNÇÕES DE UMA VEZ POR TODAS: Como resolver Funções | Resumo de Matemática para o Enem

Relasi dan Fungsi | Matematika SMP

Kurikulum Merdeka Matematika Kelas 8 Bab 4 Relasi dan Fungsi

Algebra Basics: What Are Functions? - Math Antics
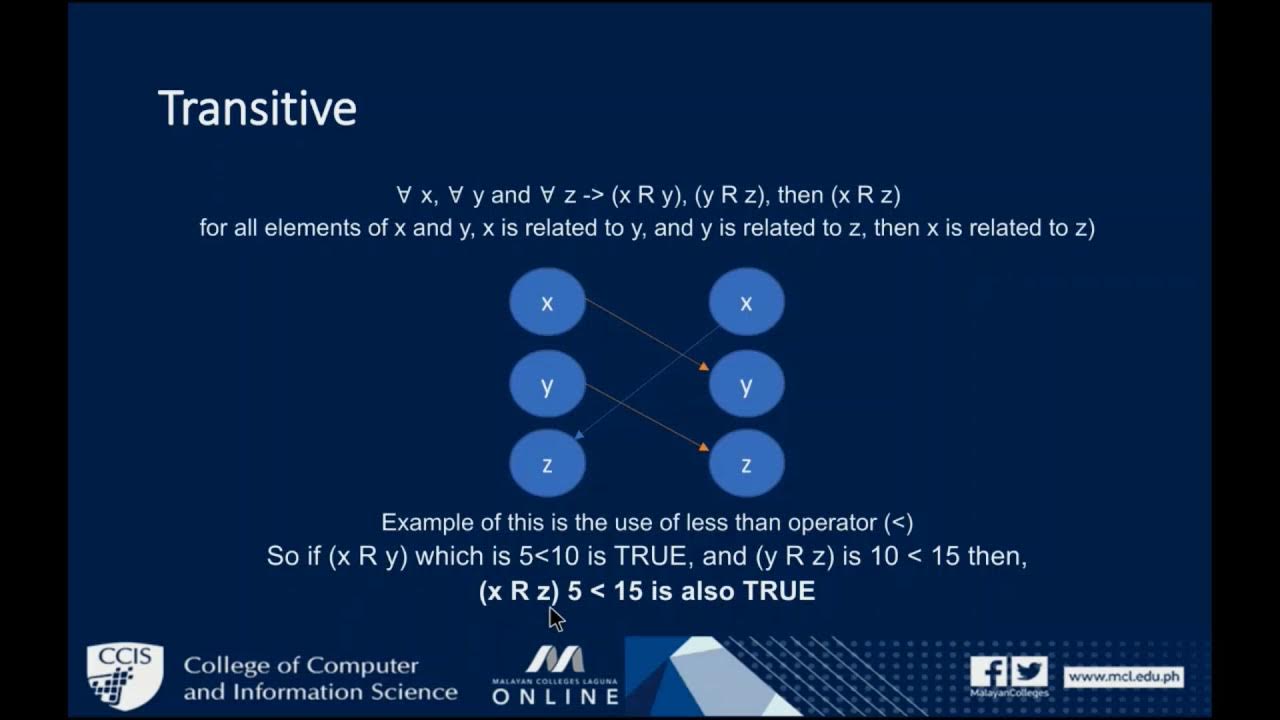
CS101 2 Module 3 Relations and their Properties PART1

Função 04: Domínio, Contradomínio e Imagem
5.0 / 5 (0 votes)
