Clock Aptitude Reasoning Tricks & Problems - Finding Angle Between The Hands of a Clock Given Time
Summary
TLDRThis video script offers a detailed explanation on calculating the angle between the hour and minute hands of an analog clock at different times. It covers various examples, such as 12:30, 1:20, 11:15, and 10:25, illustrating the step-by-step process to determine the angles. The script also explains how to find the shortest angle and the longer angle by subtracting from 360 degrees, providing a comprehensive guide for understanding clock angles.
Takeaways
- 🕒 The angle between the hour and minute hands of a clock can be calculated using the positions of the hands relative to the clock's numbers.
- 🔢 Each hour on the clock represents an angle of 30 degrees, as the full circle of 360 degrees is divided by the 12 hours.
- 📏 At 12:30, the minute hand is at the 6, and the hour hand is halfway between 12 and 1, creating an angle of 15 degrees from the 12 o'clock position.
- 📉 To find the angle at 12:30, add the angle from the 12 to the 6 (150 degrees) and the angle from the 12 to the halfway point between 12 and 1 (15 degrees), totaling 165 degrees.
- 📍 For the time 1:20, the hour hand is between 1 and 2, and the minute hand is at the 4, creating an angle that can be calculated by fractions of the hour.
- 📈 The position of the hour hand relative to the hour marks is found by dividing the minute value by 60, which gives the fraction of the hour passed.
- 🔄 At 1:20, the hour hand is two-thirds of the way between 1 and 2, and one-third of the way from 1 to 2, which translates to angles of 20 degrees and 40 degrees respectively.
- 📐 The total angle at 1:20 is found by adding the angles between the hour marks and the fractions of those angles, resulting in 80 degrees.
- 🕘 For 11:15, the minute hand is at the 3, and the hour hand is one-fourth of the way from 11 to 12, leading to an angle calculation involving fractions of 30 degrees.
- 🔢 Calculating the angle at 11:15 involves determining the fraction of the hour passed (one-fourth) and using it to find a partial angle from the 11 o'clock position (22.5 degrees).
- 📉 The total angle at 11:15 is the sum of the angle from the 12 to the 11 (90 degrees) and the partial angle (22.5 degrees), equaling 112.5 degrees.
- 🕒 For 10:25, the shortest angle between the hour and minute hands is found by considering the positions and calculating the smaller of the two possible angles, resulting in 162.5 degrees.
Q & A
What is the angle between the minute hand and the hour hand at 12:30?
-At 12:30, the minute hand is at the 6, and the hour hand is halfway between 12 and 1. Since each hour represents 30 degrees, the angle between 12 and 1 is 30 degrees. Being halfway, the angle is 15 degrees. Adding the 150 degrees from 12 to 6 gives a total angle of 165 degrees.
How do you calculate the angle for the time 1:20 on an analog clock?
-At 1:20, the minute hand is at the 4, and the hour hand is between 1 and 2. The hour hand is one-third of the way from 1 to 2. Each hour represents 30 degrees, so the angle between 1 and 2 is 30 degrees. Two-thirds of 30 degrees is 20 degrees. Adding the 30 degrees between 1 and 3 gives a total angle of 80 degrees.
What is the method to find the angle between the hour hand and the minute hand at 11:15?
-At 11:15, the minute hand is at the 3, and the hour hand is between 11 and 12. The hour hand is one-fourth of the way from 11 to 12. Each hour represents 30 degrees, so the angle between 11 and 12 is 30 degrees. Three-fourths of 30 degrees is 22.5 degrees. Adding the 90 degrees between 12 and 3 gives a total angle of 112.5 degrees.
How do you determine the shortest angle between the hour hand and the minute hand at 10:25?
-At 10:25, the minute hand is at the 5, and the hour hand is between 10 and 11. The hour hand is five-twelfths of the way from 10 to 11. Each hour represents 30 degrees, so the angle between 10 and 11 is 30 degrees. Five-twelfths of 30 degrees is 12.5 degrees. The angle between the hour hand and the minute hand is less than 180 degrees, which is 162.5 degrees.
What is the longest angle between the hour hand and the minute hand at 10:25?
-The longest angle at 10:25 is the supplementary angle to the shortest angle of 162.5 degrees, which can be found by subtracting the shortest angle from 360 degrees. So, the longest angle is 360 - 162.5 = 197.5 degrees.
How can you find the angle between the hour hand and the minute hand if the clock says 2:40?
-At 2:40, the minute hand is at the 8, and the hour hand is between 2 and 3. The hour hand is two-thirds of the way from 2 to 3. Each hour represents 30 degrees, so the angle between 2 and 3 is 30 degrees. Two-thirds of 30 degrees is approximately 20 degrees. Adding the 60 degrees between 12 and 2 gives a total angle of 80 degrees.
What is the significance of dividing the minutes by 60 when calculating the angle between the hour and minute hands?
-Dividing the minutes by 60 gives you the fraction of the hour that has passed. This fraction is used to determine how far the hour hand has moved from the last hour mark towards the next one, which is essential for calculating the angle between the hour and minute hands.
Why is it important to consider the shortest angle between the hour and minute hands?
-Considering the shortest angle is important because it represents the actual visual angle between the two hands on the clock face. It is often the most relevant measurement for practical purposes, such as determining the time until the next hour.
How does the position of the hour hand change as time passes?
-The hour hand moves continuously as time passes, covering 30 degrees for each hour. It moves at a slower pace than the minute hand, and its position relative to the hour marks changes as the minutes increase.
Can you provide a formula to calculate the angle between the hour and minute hands at any given time?
-Yes, the formula to calculate the angle between the hour and minute hands is: (hour * 30) + (minute / 2) for the hour hand, and (minute * 6) for the minute hand. The absolute difference between these two values gives the angle in degrees.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

CAT exam preparation videos 2024 |Time & Distance clocks 1 | | Quantitative Aptitude

First Grade Math Lesson Time To The Half Hour On An Analog Clock

How to say the time in English

時計算の基本【中学受験・SPI・公務員試験対策】(時計算1基本編)
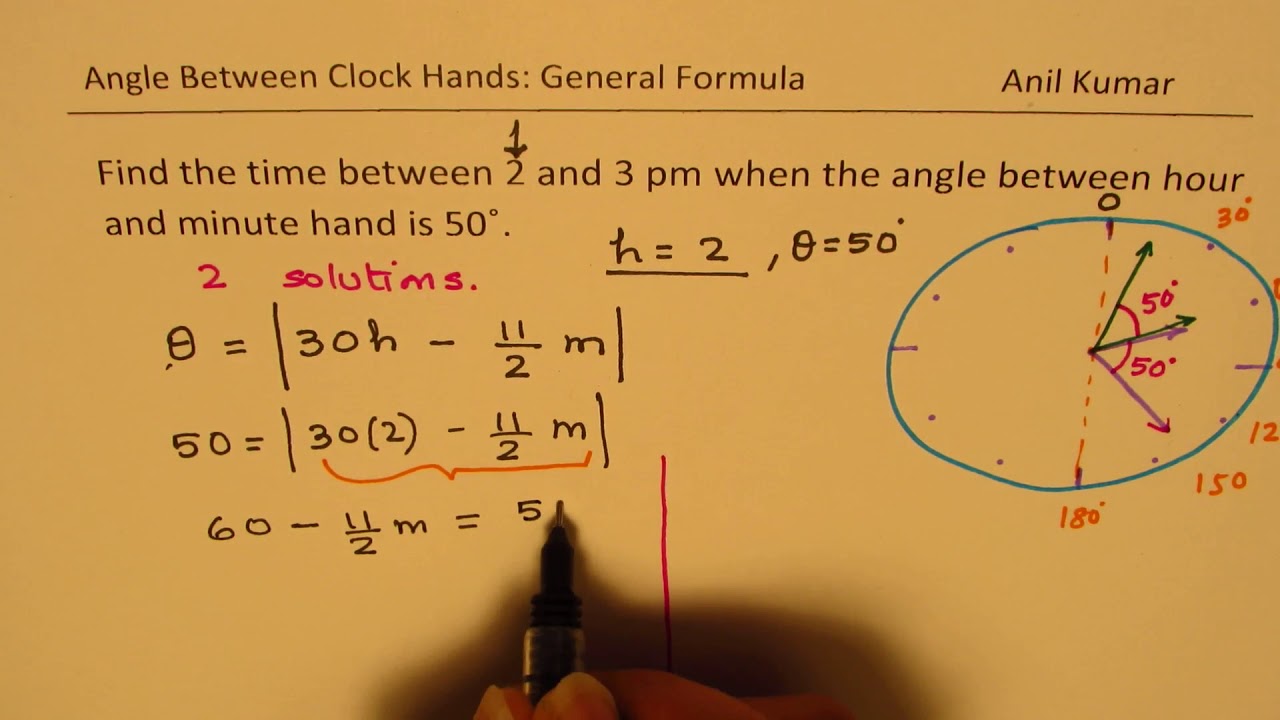
Find the time between 2 and 3 when angle is 50 between hour and minute hands
What is an Analog Signal?
5.0 / 5 (0 votes)
