TEOREMA DE PITÁGORAS | TEORIA E EXERCÍCIOS
Summary
TLDRThis lesson focuses on the Pythagorean Theorem and its applications in real-world problems. Through engaging examples, the video demonstrates how to calculate the hypotenuse of right triangles, find areas of rectangles and trapezoids, and tackle complex geometric challenges. The instructor explains each problem step-by-step, emphasizing the importance of understanding the relationship between sides in right triangles. The lesson also includes several challenges, encouraging viewers to practice and apply the theorem in diverse scenarios, enhancing their problem-solving skills in geometry.
Takeaways
- 😀 The Pythagorean Theorem states that the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides in a right triangle.
- 😀 In a right triangle, the hypotenuse is always opposite the 90-degree angle, and the other two sides are the legs (or catheti).
- 😀 To solve for the hypotenuse, use the formula: hypotenuse² = leg1² + leg2².
- 😀 When given the diagonal of a rectangle and one of its sides, you can apply the Pythagorean Theorem to find the missing side, which then helps in calculating the area of the rectangle.
- 😀 Always remember that for Pythagorean Theorem questions, the correct units (like cm² for area) should be applied after solving for the unknown side.
- 😀 For example, in a problem involving two buildings, you can use the Pythagorean Theorem to find the height of the taller building, based on the horizontal distance between them and the given diagonal.
- 😀 Be mindful of tricky wording in problems that might ask for area (e.g., the area of a rectangle) after calculating side lengths using the theorem.
- 😀 In challenges with trapezoids, use the Pythagorean Theorem to find missing lengths by creating right triangles from the given shapes.
- 😀 When a rectangle is folded into a triangle (like a paper-folding scenario), the Pythagorean Theorem can be used to calculate unknown distances, such as the diagonal.
- 😀 Common Pythagorean triplets, like (3, 4, 5), can make calculations easier and help you recognize patterns in right triangles.
Q & A
What is the Pythagorean theorem, and how is it applied?
-The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides (the catheti). It is applied by using the formula: hypotenuse² = cateto1² + cateto2².
How do you calculate the hypotenuse using the Pythagorean theorem?
-To calculate the hypotenuse, you square the lengths of the two catheti, sum them, and then take the square root of the result. For example, if one cateto is 3 cm and the other is 4 cm, the calculation would be √(3² + 4²) = √(9 + 16) = √25 = 5 cm.
What is the area of a rectangle when given its diagonal and height?
-The area of a rectangle can be calculated by multiplying its base by its height. If the diagonal and height are given, the missing base can be found using the Pythagorean theorem. Once the base is known, you can then calculate the area by multiplying base × height.
How do you find the value of X when solving for the diagonal of a rectangle?
-You can use the Pythagorean theorem by treating the diagonal as the hypotenuse and the height and base as the catheti. Once you have the values for the height and diagonal, you can solve for the missing dimension (X).
What should you do if a question asks for the area of a rectangle but gives the diagonal and height?
-First, use the Pythagorean theorem to calculate the missing side (base). Then, multiply the base by the height to find the area.
In the example involving two towers, how do you find the height of the larger tower?
-You use the Pythagorean theorem to form a right triangle, where the distance between the two towers and the known height of the smaller tower are used as catheti. The diagonal between the two points forms the hypotenuse. After calculating the missing height, you add it to the known height of the smaller tower to get the total height of the larger tower.
How do you solve for the distance X in a rectangle folded in half?
-When folding a rectangle, the resulting shape forms a right triangle. You can apply the Pythagorean theorem to the triangle, where the base and height of the rectangle become the catheti. Solving for the hypotenuse will give the required distance.
What happens when a rectangle is folded, and one corner is brought inside?
-When you fold a rectangle and bring one corner inside, it creates a right triangle with the folded portion. The length of the diagonal or the hypotenuse can be found using the Pythagorean theorem, and this helps determine the missing side (X).
What formula is used to calculate the area of a trapezoid, and how is it applied?
-The formula for the area of a trapezoid is: Area = (Base1 + Base2) / 2 × Height. You need the lengths of both bases and the height. If the base lengths are not given directly, they may need to be calculated using the Pythagorean theorem.
How do you calculate the larger base of a trapezoid when one side is unknown?
-You can calculate the larger base by using the Pythagorean theorem in the right triangle formed by dropping a perpendicular from the top base to the bottom base. Once you calculate the missing side, add it to the given base to find the total length of the larger base.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes
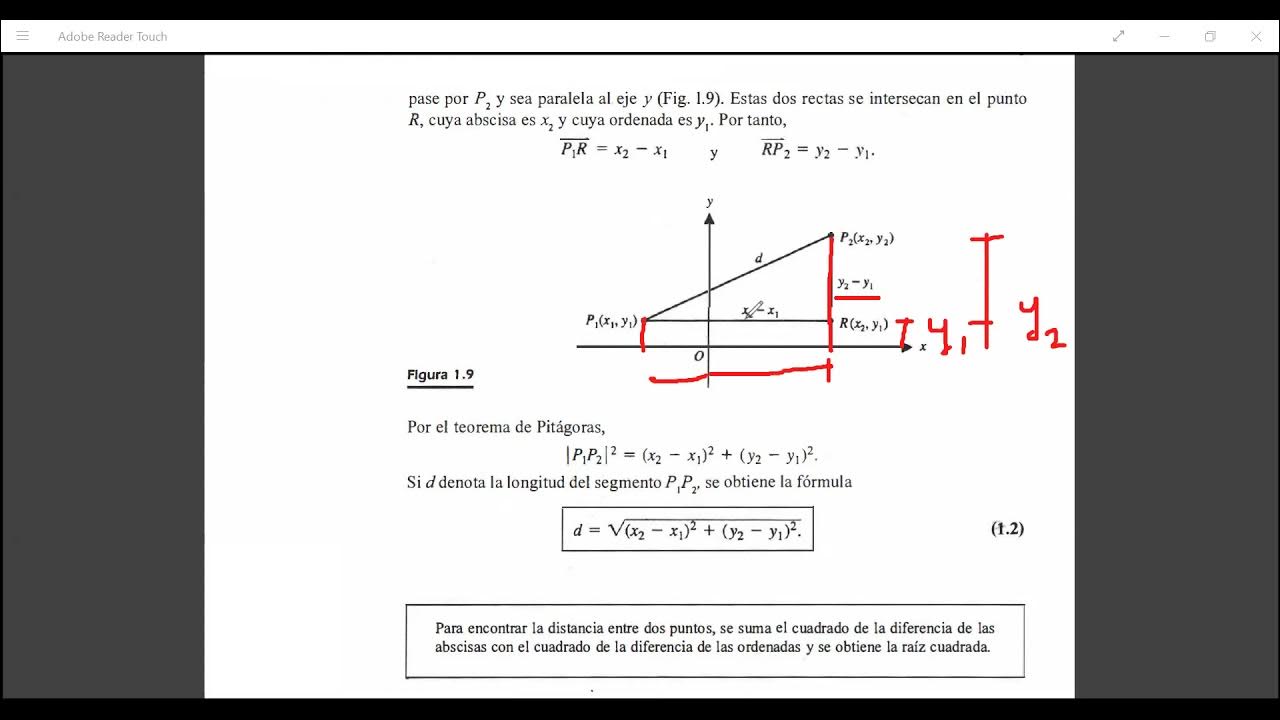
Distancia entre dos puntos
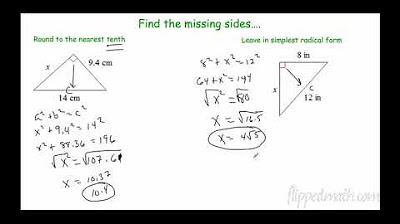
Geometry – 7.1 Pythagorean Theorem and Its Converse

Teorema Pythagoras [Part 1] - Menentukan Panjang Salah Satu Sisi Pada Segitiga Siku-siku

Physical Sciences Grade 11 – VirtualX MasterClass | Course Module 1 – Lesson 1

Introdução ao Teorema de Pitágoras | Geometria | Khan Academy
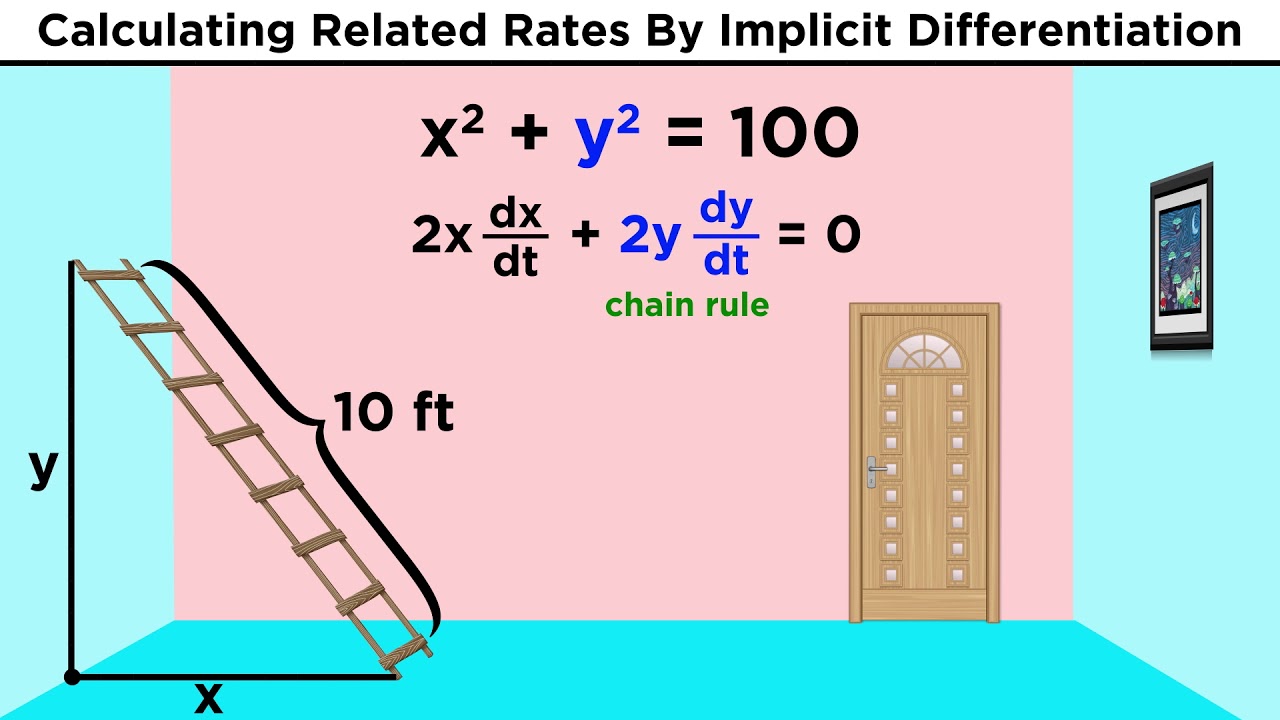
Related Rates in Calculus
5.0 / 5 (0 votes)
