Fisika Kelas 10 || Energi Mekanik dan Hukum Kekekalan Energi
Summary
TLDRThis transcript focuses on explaining the concept of mechanical energy, including the formulas for potential energy (mgh) and kinetic energy (1/2mv²). It explores energy conservation, emphasizing that energy cannot be created or destroyed, only transformed. The script also includes problem-solving examples involving objects moving along various paths, where mechanical energy remains constant. Through a series of examples, it illustrates how to calculate speed and energy changes when an object moves between points at different heights or conditions, applying principles of energy conservation and providing step-by-step solutions to physics problems.
Takeaways
- 😀 Mechanical energy is the sum of potential energy (mgh) and kinetic energy (1/2 mv^2).
- 😀 Potential energy is calculated using the formula mgh, while kinetic energy is 1/2 mv^2.
- 😀 The total mechanical energy at any point remains constant in the absence of friction or other energy losses.
- 😀 Energy can change forms but cannot be created or destroyed, as stated by the law of conservation of energy.
- 😀 In a scenario where an object moves along a path, its mechanical energy at any point is conserved and the same at different positions.
- 😀 When analyzing energy at the highest point of motion (where velocity is zero), potential energy equals mechanical energy.
- 😀 If the velocity at a certain point (e.g., point C) is zero, the object only has potential energy, and kinetic energy is zero.
- 😀 In a case where kinetic energy at point B is twice the potential energy at the same point, the object’s height from the ground can be calculated using energy conservation.
- 😀 The speed of an object at its lowest point (e.g., at point B) can be determined using the formula √(2gh), assuming no friction.
- 😀 In problems involving inclined planes, energy conservation can also be used to determine the velocity at various points based on the object's initial speed and height.
Q & A
What is mechanical energy, and how is it calculated?
-Mechanical energy is the sum of potential energy and kinetic energy. It is calculated using the formula: E_mechanical = E_potential + E_kinetic, where E_potential = mgh (mass × gravity × height) and E_kinetic = 1/2 m v^2 (half the mass multiplied by the velocity squared).
What is the law of conservation of energy?
-The law of conservation of energy states that energy cannot be created or destroyed, only transformed from one form to another. In the context of mechanical energy, it remains constant as an object moves, with potential and kinetic energy changing forms.
How can mechanical energy be the same at different points of an object's motion?
-Mechanical energy remains constant at different points of an object's motion if no external forces like friction act on the object. For example, in a system with no friction, the total mechanical energy at point A equals the total mechanical energy at point C.
In the problem, what happens when the object reaches point C with zero velocity?
-At point C, where the velocity is zero, all the mechanical energy is converted into potential energy, and the kinetic energy is zero. This happens when the object has reached the highest point of its trajectory.
What is the formula for calculating the speed at the lowest point of an object’s motion?
-The speed at the lowest point can be calculated using the formula: v = √(2gh), where g is the acceleration due to gravity and h is the height from which the object falls.
How is the height of point B determined in the second problem of the script?
-The height at point B is determined using the fact that the kinetic energy at point B is twice the potential energy. By using the conservation of mechanical energy and applying the relationship between kinetic and potential energies, the height is found to be 30 cm.
How is mechanical energy conserved in the case of a block sliding down a frictionless incline?
-As the block slides down the frictionless incline, its potential energy at the top is converted into kinetic energy as it reaches the bottom. The total mechanical energy (sum of potential and kinetic energy) remains constant throughout the motion.
What is the significance of the equation involving energy conservation in the fourth problem?
-The equation used in the fourth problem represents the conservation of mechanical energy, showing that the sum of kinetic and potential energy at point A is equal to the sum at point B. This allows the calculation of the speed at point B, where the object moves along a frictionless path.
What does the formula v = √(2gh) represent in the context of the script?
-The formula v = √(2gh) calculates the velocity of an object just before it reaches the lowest point of its fall, assuming no air resistance and that the object starts from rest.
In the last problem, how is the velocity at point B determined?
-The velocity at point B is determined by applying the principle of conservation of energy. Using the known values for height and the gravitational constant, the formula is solved for the velocity at point B, which turns out to be 8 m/s.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

Remedial fisika
Work and Energy | Grade 8 Science DepEd MELC Quarter 1 Module 3 Part 2 Potential Energy

Belajar IPA - Energi Kinetik, Potensial dan Mekanik Kelas 7 SMP/MTs #Energi #energikinetik

Energia Cinética, Potencial Gravitacional e Potencial Elástica

Conservation of Mechanical Energy | Physics
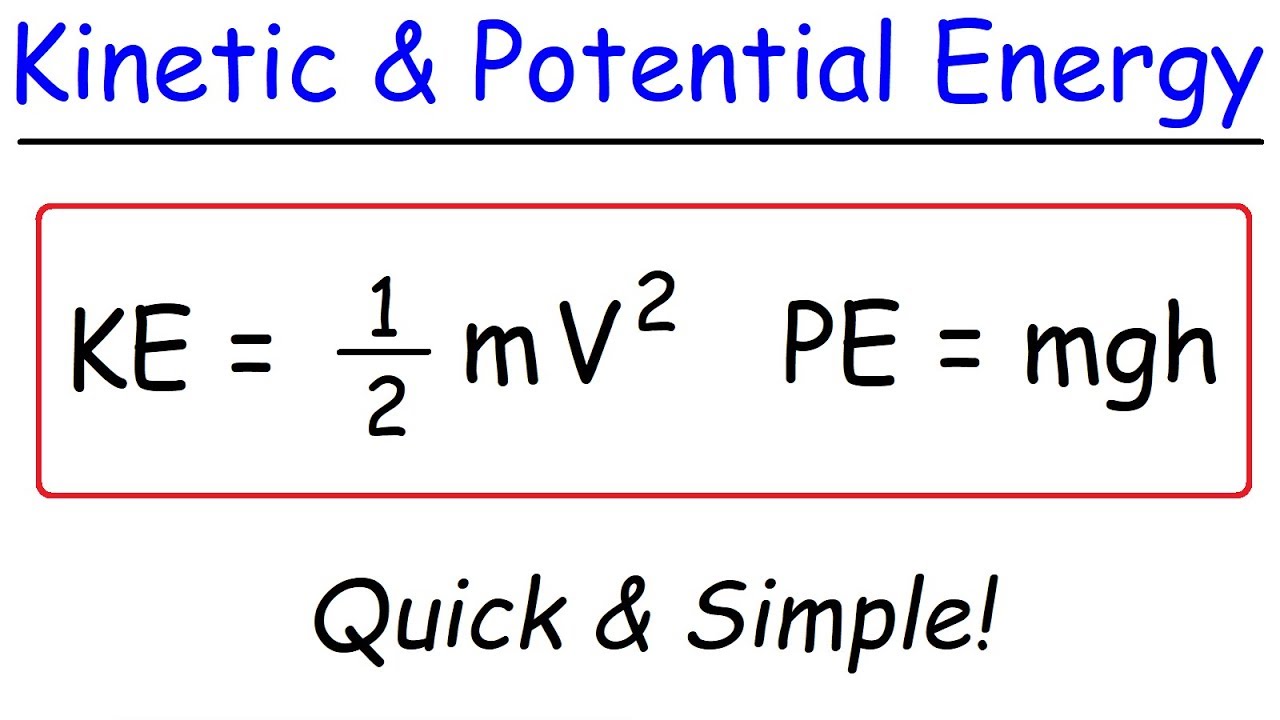
Kinetic Energy and Potential Energy
5.0 / 5 (0 votes)
