INTRODUÇÃO AO CURSO DE INTEGRAL ✅ INTEGRAL INDEFINIDA #02

Summary

This lesson covers key concepts in integral calculus, focusing on the primitive functions of common mathematical expressions. The instructor explains the importance of understanding basic functions such as sine, cosine, and power functions, and how to derive their primitives. They also discuss essential properties of primitive functions, like the sum and multiplication by a constant. Examples are provided, including how to calculate the primitive of polynomial and trigonometric functions. The session emphasizes the verification of results through differentiation to ensure the accuracy of the obtained primitives.

Takeaways

• 😀 The primitive (antiderivative) of a function is a key concept in calculus, where the goal is to reverse the derivative process.
• 😀 The derivative of an exponential function like e^(x+c) is e^x, with the constant 'c' becoming irrelevant in the derivative.
• 😀 The derivative of sine is cosine, and the derivative of cosine is negative sine, which requires careful attention to signs.
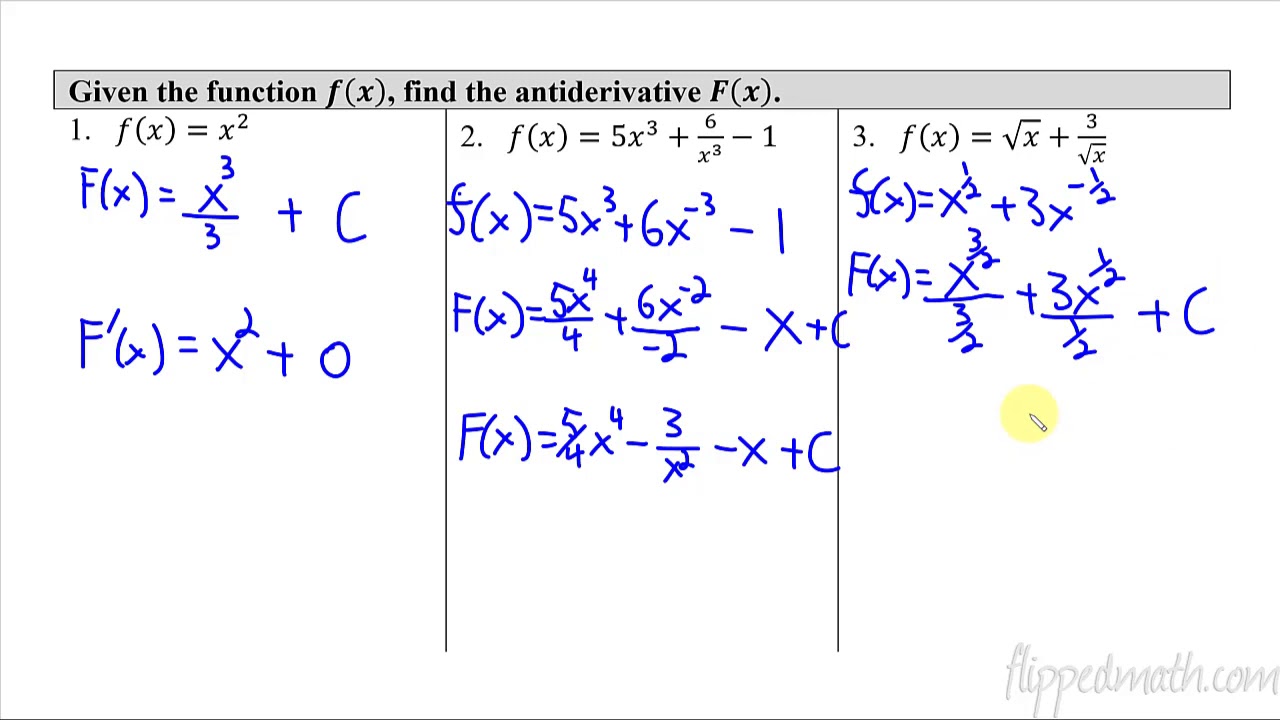
• 😀 The antiderivative of a power function x^n is (x^(n+1)) / (n+1), as long as n is not equal to -1.
• 😀 If you have a constant factor multiplied by a function, the constant can be factored out when computing the antiderivative.
• 😀 The antiderivative of x^2 is (x^3) / 3, and similarly, higher powers of x follow this rule of adding 1 to the exponent and dividing by the new exponent.
• 😀 The antiderivative of a constant (like 2) is simply the constant times x, which can be calculated directly.
• 😀 The antiderivative of 1/x is the natural logarithm (ln|x|), with the modulus of x ensuring the argument inside the log is positive.
• 😀 When integrating sums of functions, you can apply the antiderivative to each function individually and then sum the results.
• 😀 In problems involving trigonometric functions, the derivative of sine is cosine, and the derivative of cosine is negative sine, so care must be taken with signs when deriving expressions involving trigonometric functions.

Q & A

What is the concept of the 'primitive' or 'antiderivative' discussed in the transcript?

The 'primitive' or 'antiderivative' of a function refers to the reverse process of differentiation. It is the function whose derivative gives the original function. In the transcript, it was explained that if you derive the function e^x, you get e^x, and similarly for trigonometric functions like sine and cosine.

Why is the constant 'c' important when discussing primitives?

The constant 'c' is essential because the primitive of a function can have an infinite number of solutions, each differing by a constant. This is why the general form of a primitive function includes the constant 'c', which represents all possible shifted versions of the function.

What happens when you derive e^x + c?

When you derive e^x + c, the result is e^x. This is because the derivative of e^x is e^x, and the derivative of a constant (c) is 0, leaving e^x as the result.

How do you calculate the primitive of a power function, such as x^8?

To calculate the primitive of x^8, you increase the exponent by 1, making it x^9, and then divide by the new exponent (9). Therefore, the primitive of x^8 is x^9/9.

What is the result when deriving the function x^9?

When you derive x^9, the result is 9x^8. This is due to the power rule of differentiation, which brings down the exponent as a multiplier and then reduces the exponent by 1.

How do you handle a constant multiplying a function when calculating its primitive?

When a constant is multiplying a function, you can factor the constant out of the primitive calculation. For example, if you have 5x^3, the constant 5 can be factored out, and you would then calculate the primitive of x^3, which would be x^4/4. The final result would be 5x^4/4.

What is the primitive of the function 1/x?

The primitive of the function 1/x is the natural logarithm of the absolute value of x, denoted as ln|x|, plus a constant. This is because the derivative of ln|x| is 1/x.

How can you check if a given result is correct when calculating a primitive?

You can check if the result of a primitive is correct by differentiating it. If the derivative of the result matches the original function, then the calculation is correct.

What is the importance of applying the properties of primitives, such as linearity and constants, during calculations?

The properties of primitives, like linearity (the primitive of a sum is the sum of the primitives) and handling constants, simplify the process of calculating complex integrals. For example, when dealing with multiple terms, you can compute the primitive of each term individually and then sum them up.

What does it mean when the derivative of a function results in a negative sign, such as when differentiating cosine?

When you differentiate cosine, the result is negative sine. This is a standard result from calculus: the derivative of cos(x) is -sin(x). The negative sign appears because of the inherent properties of the cosine and sine functions in calculus.