Standing Waves
Summary
TLDRThis video explains the concept of standing waves, particularly in the context of stringed instruments like violins and guitars. It covers rigid boundary conditions, where the medium is fixed at both ends, creating nodes and antinodes. The speaker discusses harmonics, the relationship between frequency and wavelength, and how the length, tension, and mass density of a string affect the pitch. The fundamental frequency, harmonic series, and methods of altering pitch are explored. The video also briefly touches on free boundary conditions, where the ends of a string move freely, in contrast to the fixed points seen in most stringed instruments.
Takeaways
- 😀 Standing waves are a key concept in introductory physics, often demonstrated in stringed instruments like guitars, violins, and harps.
- 😀 Standing waves occur when a medium is constrained at both ends, forcing the wave to fit within these fixed points.
- 😀 In rigid boundary conditions, the medium is pinned down at both ends, preventing movement at the boundaries, but allowing vibration in the middle.
- 😀 Nodes are points where the wave experiences no displacement, while anti-nodes are points where the wave experiences maximum displacement.
- 😀 Harmonics are formed as the string vibrates, and each harmonic corresponds to a different frequency based on the wave's pattern.
- 😀 The fundamental frequency is the lowest frequency in the harmonic series, where the string vibrates with half the wavelength fitting within the string.
- 😀 The harmonic series is formed by integer multiples of the fundamental frequency (e.g., 2f1, 3f1, etc.).
- 😀 The string's length directly affects the frequency; longer strings produce lower frequencies (deeper tones), while shorter strings produce higher frequencies (higher-pitched tones).
- 😀 Tension in the string increases the wave's speed, raising the frequency and pitch, while a lower tension decreases the pitch.
- 😀 Mass density (the thickness of the string) influences the frequency, with thicker strings resulting in lower frequencies and deeper tones.
- 😀 Free boundary conditions allow the medium to move freely at the ends, unlike rigid boundary conditions where the ends are fixed in place.
Q & A
What are standing waves and why are they important in physics?
-Standing waves are waves that remain in a constant position, oscillating between fixed points, and they are crucial because they represent wave patterns seen in musical instruments like harps, violins, and guitars. They were among the first types of waves studied in physics.
What defines the boundary conditions for standing waves?
-The boundary conditions for standing waves depend on whether the boundaries are rigid or free. In rigid boundary conditions, the ends of the medium are fixed, preventing movement, while in free boundary conditions, the ends can move freely.
What happens at a rigid boundary in the context of standing waves?
-At a rigid boundary, the medium is fixed, preventing any movement at the ends. The string or medium can vibrate freely between these fixed ends, and only certain wavelengths that fit between these boundaries are allowed.
What are nodes and anti-nodes in standing waves?
-Nodes are points where there is no displacement, and they remain stationary during the wave's oscillation. Anti-nodes are points where the displacement is maximal and move the most during the wave's motion.
How do you determine the wavelength for different harmonics in standing waves?
-The wavelength for different harmonics in standing waves can be determined by counting the number of nodes and anti-nodes. For example, for the first harmonic, the wavelength is twice the length of the string, and for the second harmonic, the wavelength is equal to the length of the string.
What is the relationship between the frequency and the harmonic number in standing waves?
-The frequency of the nth harmonic is given by the formula f_n = n * f_1, where n is the harmonic number and f_1 is the fundamental frequency. This creates a harmonic series where each frequency is an integer multiple of the fundamental frequency.
What is the effect of changing the length of the string on the fundamental frequency?
-Increasing the length of the string decreases the fundamental frequency, resulting in a lower pitch, while decreasing the string's length increases the fundamental frequency, producing a higher pitch.
How does tension in a string affect the speed of a wave and its frequency?
-Increasing the tension in the string increases the speed of the wave, which in turn raises the fundamental frequency, making the sound higher pitched. This can be observed in musical instruments where tuning adjusts tension to change pitch.
What role does mass density play in the frequency of standing waves?
-The mass density of the string affects the wave speed, with higher mass density resulting in a slower wave speed and thus a lower fundamental frequency. This is why thicker strings, which have greater mass density, produce lower pitches.
What are free boundary conditions and how are they different from rigid boundary conditions?
-Free boundary conditions occur when the ends of the medium are not fixed but can move freely. This contrasts with rigid boundary conditions, where the ends are fixed and cannot move. Free boundary conditions are more commonly seen in sound waves, whereas rigid boundary conditions are typical for stringed instruments.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

Gelombang Bunyi Pada Dawai (Senar) | Materi Fisika Gelombang Bunyi Kelas 11 SMA
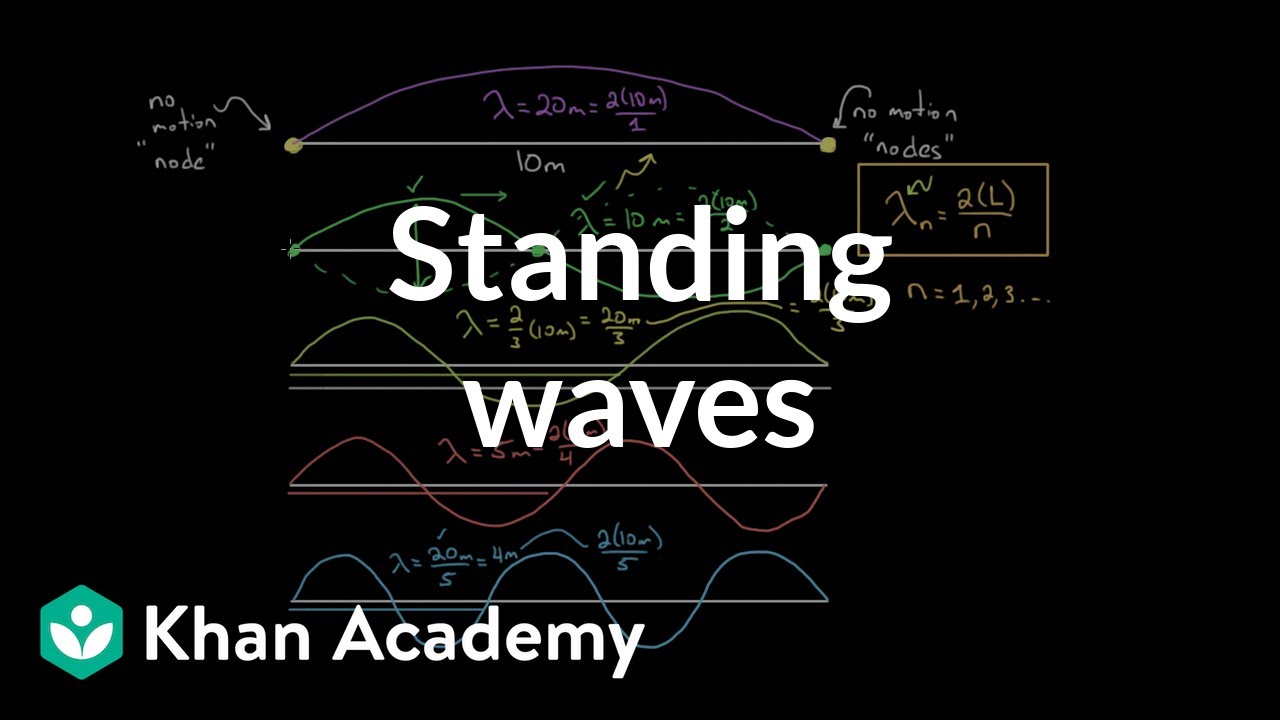
Standing waves on strings | Physics | Khan Academy

Cremona – The Italian City of Violins | Full Documentary

The Physics of Music: Crash Course Physics #19

Gelombang Bunyi • Part 3: Sumber Bunyi (Dawai, Pipa Organa Terbuka dan Tertutup)
DAWAI (SENAR) SEBAGAI SUMBER BUNYI | Gelombang Bunyi #1 - Fisika Kelas 11
5.0 / 5 (0 votes)
