Dinamika Rotasi • Part 1: Momen Gaya / Torsi
Summary
TLDRIn this video, Christian Sutantio explains the concept of rotational dynamics for high school students, focusing on the moment of force (torque). He covers the definition and importance of torque in rotational motion, how it differs from linear force, and the necessary formulas for calculating it. The video also discusses how to handle cases where the applied force is not perpendicular to the lever arm, with step-by-step techniques to resolve such forces. Examples are given to demonstrate the practical application of these concepts, making it a thorough guide to understanding rotational motion in physics.
Takeaways
- 😀 The video covers the topic of rotational dynamics, specifically focusing on the concept of torque (moment of force) in physics.
- 😀 Torque is explained as the tendency of a force to rotate an object around a fixed axis, unlike linear force which causes straight-line motion.
- 😀 The difference between force and torque is emphasized, with torque causing rotational motion and force causing linear motion.
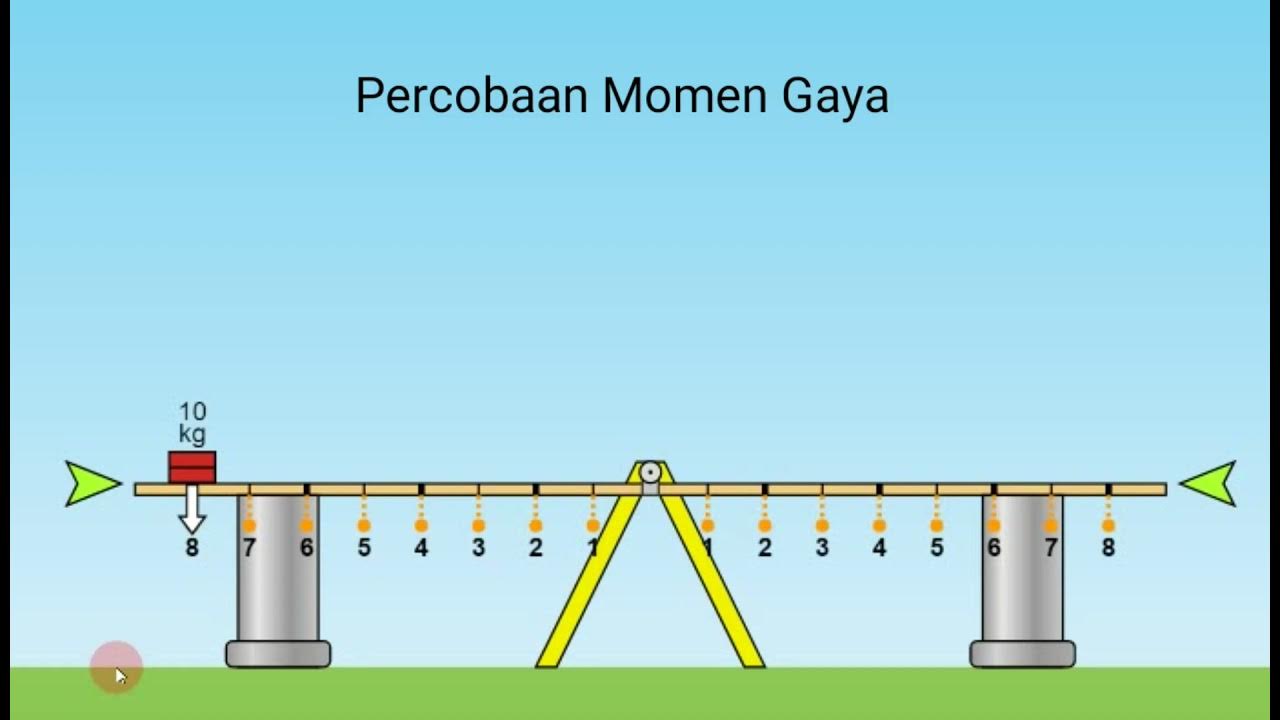
- 😀 The formula for calculating torque (τ = F * r) is introduced, where τ is torque, F is force, and r is the perpendicular distance from the axis of rotation.
- 😀 Torque is defined as positive if it causes counterclockwise rotation and negative if it causes clockwise rotation.
- 😀 The video explains how to calculate torque when the force is not perpendicular to the lever arm, and introduces two methods for solving such problems: using trigonometric components or extending the force to form a right angle.
- 😀 Method 1 involves resolving the force into its components and using the perpendicular component to calculate the torque.
- 😀 Method 2 involves extending the force line to form a right triangle with the axis of rotation, ensuring that the perpendicular distance is used for torque calculation.
- 😀 The video emphasizes that both methods lead to the same result, but some situations may make one method more practical than the other.
- 😀 An example problem is worked through where the torque is calculated at different points along a rotating object using the methods discussed, demonstrating the application of the formulas and the concepts of positive and negative torque.
Q & A
What is the difference between force and torque?
-Force causes an object to move in a straight line, while torque causes an object to rotate around a pivot or axis. Torque is a turning force that acts on an object to make it spin, whereas force is a push or pull that moves an object along a linear path.
What is the formula for calculating torque?
-The formula for calculating torque is τ = F × r, where τ is torque, F is the force applied, and r is the distance from the pivot point to where the force is applied (the lever arm). If the force is applied at an angle, the formula becomes τ = F × r × sin(θ), where θ is the angle between the force and the lever arm.
How do we determine the direction of torque?
-The direction of torque is determined by the direction of rotation. If the rotation is clockwise, the torque is negative. If the rotation is counterclockwise, the torque is positive. This helps to define the sign of the torque when solving problems involving multiple forces.
What is the significance of the angle in the formula for torque?
-The angle (θ) in the torque formula affects how much of the applied force contributes to the rotation. Only the component of the force that is perpendicular to the lever arm generates torque. The sine of the angle (sin(θ)) gives the magnitude of the force that is perpendicular to the lever arm.
Why is the distance from the pivot point important in calculating torque?
-The distance from the pivot point, known as the lever arm (r), is crucial because the longer the lever arm, the greater the torque for the same amount of force. A longer lever arm allows for a larger turning effect, making it easier to rotate an object.
What happens when a force is applied at an angle to the lever arm?
-When a force is applied at an angle, we need to break it down into its components—one parallel and one perpendicular to the lever arm. Only the perpendicular component contributes to the torque. This is why the torque formula includes sin(θ), which accounts for the perpendicular force.
What are the two tricks used to calculate torque when the force is applied at an angle?
-The two tricks are: 1) Break the force into its horizontal and vertical components, and use the vertical component to calculate torque. 2) Extend the force to find a line perpendicular to the pivot, and use the perpendicular component of the force to calculate torque. Both methods ultimately lead to the same result.
What is the significance of the point where the force is applied when calculating torque?
-The point where the force is applied affects the length of the lever arm (r) and, therefore, the torque. If the force is applied at the pivot point, the distance is zero, and no torque is generated. The farther from the pivot the force is applied, the greater the torque.
How do we calculate the total torque when multiple forces are acting on an object?
-To calculate the total torque, we sum up the individual torques from all the forces acting on the object. Each torque is calculated based on the force, the lever arm distance, and the direction of rotation (clockwise or counterclockwise). Remember to account for the sign (positive or negative) based on the direction of rotation.
How do we determine if the torque will be positive or negative in a multi-force system?
-The direction of the torque depends on the direction of the rotation caused by each force. If the force causes a counterclockwise rotation, the torque is positive. If it causes a clockwise rotation, the torque is negative. When solving for total torque, be sure to assign the correct sign to each torque based on the rotation direction.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen
5.0 / 5 (0 votes)