😋🤤🍑🍒🍌Full Video school teacher Navel #trending #navel #teacher #teachers #school #schoolteachers

Summary

The transcript presents a comprehensive geometry lesson focused on concepts such as parallelograms, angles, and their properties. It explores the relationship between parallel lines and interior angles, providing detailed explanations of various geometric rules, including properties of diagonals in parallelograms and rectangles. The lesson emphasizes problem-solving techniques, guiding students step-by-step through calculations of unknown angles using properties like linear pairs, alternate angles, and opposite angles. The teacher also stresses the importance of practice, ensuring students understand how to apply these concepts to solve geometry problems effectively.

Takeaways

• 😀 Always focus on the given diagram and ensure all provided angles and properties are carefully noted before solving.
• 😀 Parallel lines in geometric figures often imply key properties, like the sum of interior angles being 180 degrees.
• 😀 When solving for unknown angles, utilize angle relationships such as linear pairs and opposite angles.
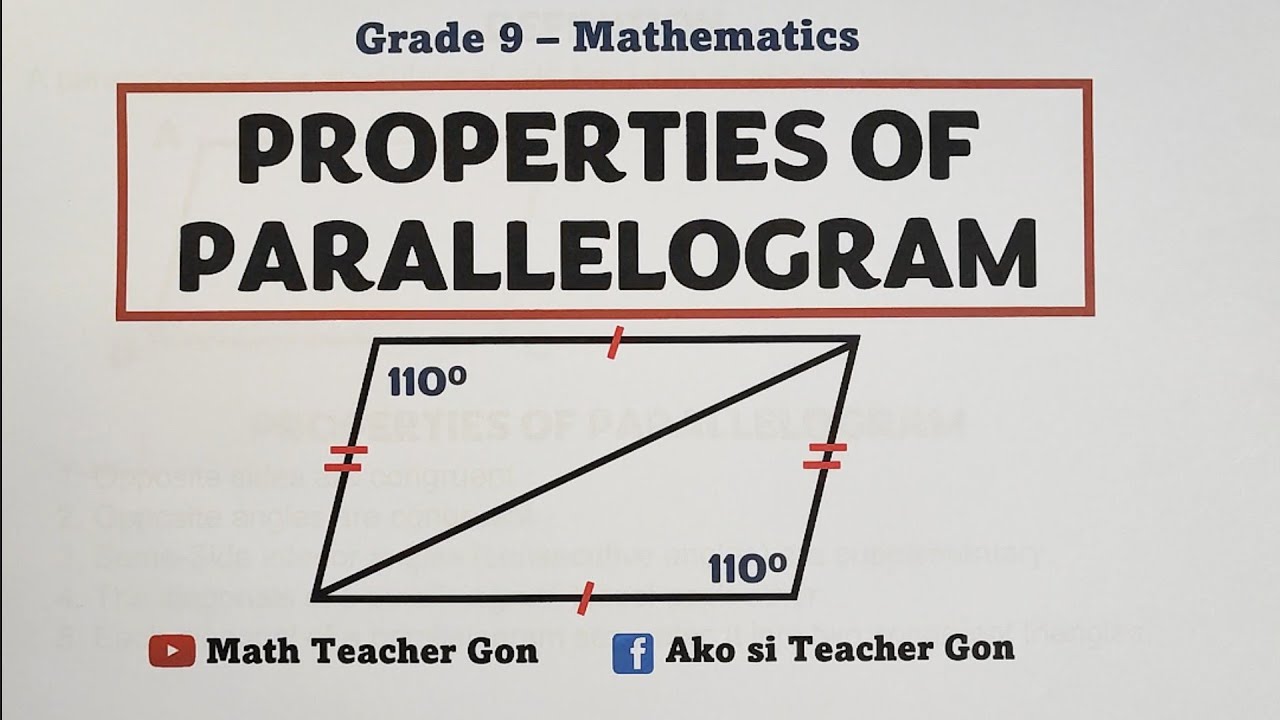
• 😀 The opposite angles of a parallelogram are equal and can be used to find missing values.
• 😀 For parallel lines intersected by a transversal, alternate interior angles are congruent.
• 😀 The sum of interior angles in a triangle always equals 180 degrees, which helps in solving for unknown angles.
• 😀 To solve for the angles in a parallelogram, the property that opposite sides are equal can be used.
• 😀 When working with diagonals of a parallelogram, remember they bisect each other but are not necessarily equal in length.
• 😀 If a quadrilateral has one pair of opposite sides parallel, it can be classified as a trapezium.
• 😀 When dealing with rectangles, recall that opposite sides are equal, and all angles are 90 degrees. The diagonals are equal and bisect each other.

Q & A

What is the first step when solving a geometry problem with parallel lines and angles?

The first step is to identify the given angles and properties of the shapes involved, such as parallel lines and opposite angles. Then, use properties like alternate interior angles and supplementary angles to find missing values.

How do you find the value of angle X in a parallelogram when one of its angles is 70°?

Since the sum of adjacent angles in a parallelogram is 180°, you can subtract the given angle (70°) from 180° to find the other angle. Therefore, angle X = 180° - 70° = 110°.

What is the property of opposite angles in a parallelogram?

In a parallelogram, opposite angles are equal. So, if angle X is 110°, the opposite angle will also be 110°.

What is the property of alternate angles when two parallel lines are cut by a transversal?

Alternate interior angles formed by two parallel lines cut by a transversal are equal. For example, if one angle is 40°, the alternate angle will also be 40°.

How do you find angle Z in a triangle where the sum of angles P and Q is 150°?

The sum of angles in a triangle is always 180°. So, to find angle Z, subtract the sum of angles P and Q from 180°. Therefore, angle Z = 180° - 150° = 30°.

What is the rule for the opposite sides of a parallelogram?

The opposite sides of a parallelogram are equal in length. For example, if one side is 18 units, the opposite side will also be 18 units.

What does it mean for the diagonals of a parallelogram?

In a parallelogram, the diagonals bisect each other. This means each diagonal divides the other into two equal parts.

What property do diagonals of a rectangle have?

In a rectangle, the diagonals are equal in length and bisect each other.

What can be concluded if two adjacent angles in a trapezium add up to 180°?

If two adjacent angles in a trapezium add up to 180°, the lines forming those angles are parallel. This is a defining property of trapeziums.

How do you determine the value of an unknown angle in a parallel line configuration?

To find an unknown angle in a parallel line configuration, you can use the property that the sum of interior angles on the same side of a transversal is 180°. For example, if one angle is given as 120°, the other will be 60° because 180° - 120° = 60°.