Integral de la exponencial | Integrales por Sustitución - Cambio de variable | Ejemplo 14
Summary
TLDRThis educational video tutorial explains the process of solving integrals by substitution, focusing on cases with exponential functions. The instructor walks viewers through identifying when substitution is applicable, particularly when the derivative of the exponent closely matches the rest of the expression. The video includes a detailed example, showing step-by-step how to transform the integral into a solvable form, and concludes with practice problems. The instructor encourages viewers to pause the video and try solving on their own before comparing answers. Overall, the tutorial aims to strengthen understanding of integration techniques and substitution.
Takeaways
- 😀 Substitution or change of variable is a technique for solving integrals, particularly when dealing with exponentials.
- 😀 The key condition for applying substitution is when the derivative of the exponent in the exponential function matches a part of the integrand.
- 😀 If you have an exponential with a variable in the exponent (e.g., e^(x^2)), and the derivative of the exponent is a factor in the integrand, substitution can be used.
- 😀 To apply substitution, identify the derivative of the exponent and check if it matches a part of the integrand to facilitate the change of variable.
- 😀 For example, in the integral of e^(x^2), the derivative of x^2 is 2x, which suggests substitution.
- 😀 When performing substitution, replace the variable and its differential (e.g., 2x dx) with a new variable (e.g., u).
- 😀 After substitution, the integral often simplifies to a more straightforward form, such as e^u du, which is easy to integrate.
- 😀 Once the integration is completed, don’t forget to return to the original variable by reversing the substitution.
- 😀 The integral of e^u with respect to u is simply e^u, and the final result should include the constant of integration.
- 😀 It’s important to ensure that you modify the integral correctly, adjusting for any missing factors or terms (like a missing '2' or '3').
- 😀 Practice and attention to detail are key in mastering substitution, as demonstrated through multiple example problems in the video.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

✅INTEGRALES trigonométricas con POTENCIAS [𝙚𝙣 4 𝙋𝙖𝙨𝙤𝙨 😎🫵💯 ] Cálculo Integral
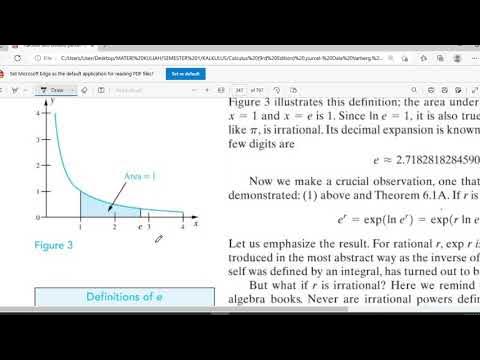
Fungsi Transenden 3 (Fungsi Eksponensial Natural)
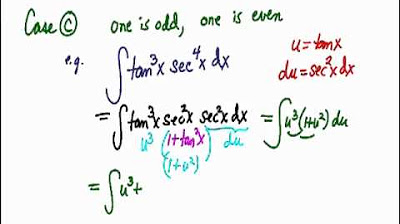
Trig Integrals Tan Sec

LENGKAP Integral tak tentu, integral tertentu, integral subtitusi dan integral parsial
Integral yang memuat bentuk kuadratik ax^2+bx+c

Integración por sustitución | Ejemplo 4 | Multiplicación
5.0 / 5 (0 votes)