Pensamiento Matemático II PROGRESION 11
Summary
TLDRIn this educational video, the presenter covers key concepts in mathematical thinking for high school students, focusing on Cartesian coordinates, calculating distances between points, and determining the area of geometric shapes. The video walks through the basics of Cartesian coordinates, including the X and Y axes, the origin, and the four quadrants. Practical examples follow, involving locating points on a coordinate plane and solving distance and area problems. The presenter also explains how to apply both analytical and synthetic methods to calculate areas, providing a comprehensive understanding of geometry. Viewers are encouraged to practice the concepts through interactive exercises.
Takeaways
- 😀 The Cartesian coordinate system consists of two perpendicular axes that intersect at the origin, with the horizontal axis being the x-axis (abscissas) and the vertical axis being the y-axis (ordinates).
- 😀 Coordinates of any point in the plane are represented as an ordered pair (x, y), where x represents the horizontal distance (along the x-axis) and y represents the vertical distance (along the y-axis).
- 😀 The Cartesian plane is divided into four quadrants, each having different signs for the x and y coordinates: Quadrant I (x and y positive), Quadrant II (x negative, y positive), Quadrant III (x and y negative), and Quadrant IV (x positive, y negative).
- 😀 To locate points on the Cartesian plane, one moves horizontally along the x-axis and then vertically along the y-axis from the origin.
- 😀 A problem is presented where a group of friends' meeting locations are mapped using coordinates. The task is to determine the coordinates of various locations based on the given description.
- 😀 The video demonstrates how to calculate the coordinates of meeting locations, such as Angélica’s (0,0), Cintia’s (3,-4), and Karina’s (4,3).
- 😀 Viewers are encouraged to practice locating points and calculating coordinates based on descriptions of positions in real-life scenarios.
- 😀 The script explains how to calculate the distance between two points using the Pythagorean theorem. The distance formula involves the square root of the sum of squared differences in the x and y coordinates of two points.
- 😀 The distance between points A(2,1) and B(5,6) is calculated using the distance formula, resulting in approximately 5.83 units.
- 😀 The video provides a practice problem where viewers calculate the distance between points A(-3,2) and B(4,-1), ultimately resulting in a distance of approximately 7.61 units.
- 😀 Lastly, the video explains how to calculate the area of geometric shapes (such as triangles) using both analytic geometry (determinant method) and synthetic geometry (Heron’s formula), demonstrating the equivalence of both methods.
Q & A
What is the Cartesian coordinate system?
-The Cartesian coordinate system consists of two perpendicular axes, typically referred to as the x-axis (horizontal) and the y-axis (vertical), which intersect at the origin. Points on the plane are represented by ordered pairs (x, y), where x is the distance along the x-axis and y is the distance along the y-axis.
What are the four quadrants of the Cartesian plane?
-The Cartesian plane is divided into four quadrants: the first quadrant (top-right) where both x and y are positive, the second quadrant (top-left) where x is negative and y is positive, the third quadrant (bottom-left) where both x and y are negative, and the fourth quadrant (bottom-right) where x is positive and y is negative.
How are points represented on the Cartesian plane?
-Points are represented by an ordered pair (x, y). The x-coordinate indicates the horizontal position (distance along the x-axis), while the y-coordinate represents the vertical position (distance along the y-axis).
What is the significance of the origin in the Cartesian plane?
-The origin, located at the intersection of the x-axis and y-axis, has coordinates (0, 0). It serves as the reference point for measuring the distance of other points in the plane.
What is the formula for calculating the distance between two points on the Cartesian plane?
-The distance formula is derived from the Pythagorean theorem and is given by: d = √[(x2 - x1)² + (y2 - y1)²], where (x1, y1) and (x2, y2) are the coordinates of the two points.
How do you calculate the distance between two points using the distance formula?
-To calculate the distance between two points, first find the differences between their x and y coordinates (x2 - x1 and y2 - y1). Then square these differences, sum them, and take the square root of the result.
What is the method for calculating the area of a triangle using the Cartesian plane?
-The area of a triangle on the Cartesian plane can be calculated using the determinant formula. The formula involves the coordinates of the triangle's vertices and is expressed as: Area = 1/2 | x1(y2 - y3) + x2(y3 - y1) + x3(y1 - y2) |.
What is Heron's formula and how is it used to calculate the area of a triangle?
-Heron's formula calculates the area of a triangle when the lengths of its sides are known. First, calculate the semiperimeter (s) as half the sum of the side lengths. Then, the area is given by the formula: Area = √[s(s - a)(s - b)(s - c)], where a, b, and c are the side lengths of the triangle.
What are the coordinates of the houses of Cintia and Karina in the given example?
-In the example, Cintia’s house is located at the coordinates (3, -4) and Karina’s house is located at (4, 3). These are derived by following the directions given in the video to move along the x and y axes from the origin.
How do you determine the coordinates of a point from the origin?
-To determine the coordinates of a point from the origin, count the horizontal (x-axis) and vertical (y-axis) distances from the origin to the point. The x-coordinate is the horizontal distance, and the y-coordinate is the vertical distance.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
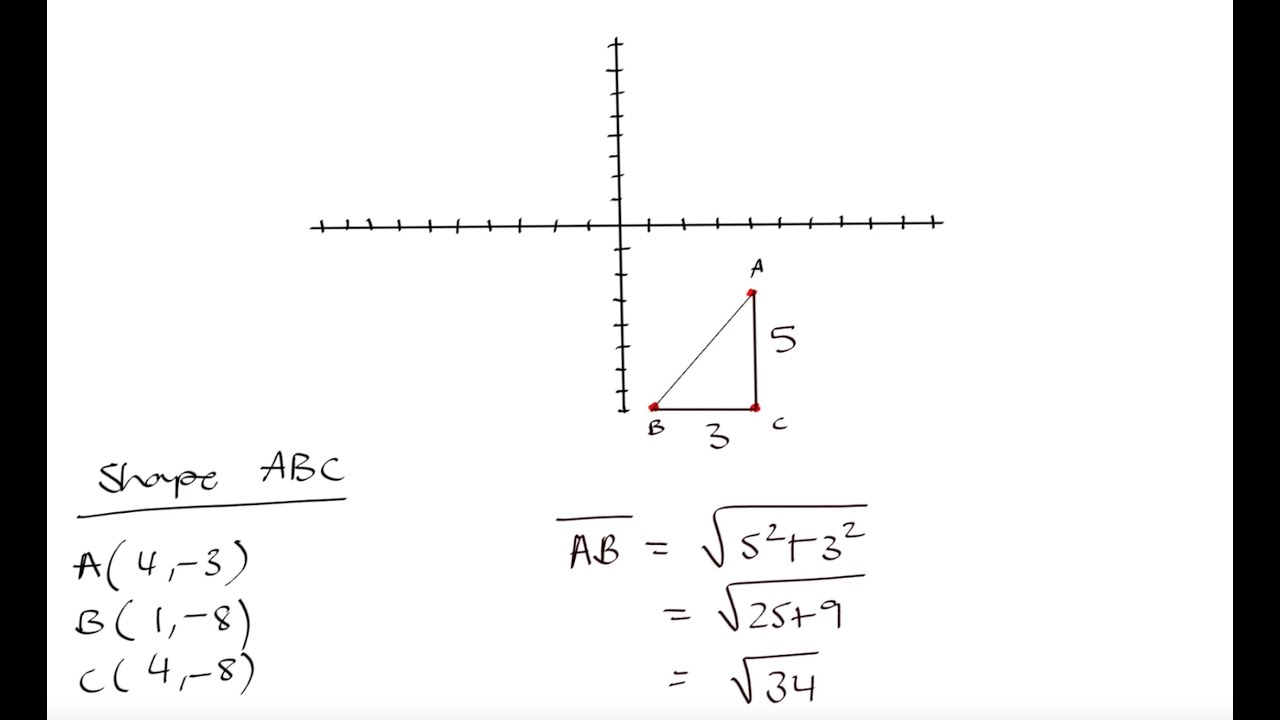
Grade 10 Math: Drawing geometric figures on a Cartesian plane

HUBUNGAN ANTARA UNSUR UNSUR DALAM BANGUN DATAR DAN BANGUN RUANG

Mengingat Statistik Hal 79-84 Bab 3 STATISTIK Kelas 11 SMA Kurikulum Merdeka

Matematika Kelas 9: Bangun Ruang Sisi Lengkung (Tabung, Kerucut, Bola)

MATEMATIKA KELAS 9 HALAMAN 137-143 KURIKULUM MERDEKA EDISI 2022
Math 5 Quarter 1 Week 6 Revised K-12 Area Of A Parallelogram, Triangle, And Trapezoid
5.0 / 5 (0 votes)